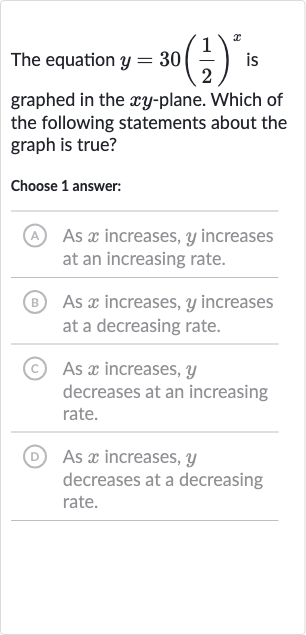
The equation y=30((1)/(2))^(x) is graphed in the xy-plane. Which of the following statements about the graph is true? Choose 1 answer: (A) As x increases, y increases at an increasing rate. (B) As x increases, y increases at a decreasing rate. (c) As x increases, y decreases at an increasing rate. (D) As x increases, y decreases at a decreasing rate.

Question

The equation  y=30((1)/(2))^(x) is graphed in the  xy-plane. Which of the following statements about the graph is true? Choose 1 answer: (A) As  x increases,  y increases at an increasing rate. (B) As  x increases,  y increases at a decreasing rate. (c) As  x increases,  y decreases at an increasing rate. (D) As  x increases,  y decreases at a decreasing rate.

Bytelearn · Accepted Answer

Given Equation Analysis: We are given the equation y = 30(1/2)^x and need to determine how y changes as x increases. The base of the exponent, 1/2, is less than 1, which indicates that we have an exponential decay function.Exponent Behavior: To understand the behavior of the graph, we can analyze the exponent. As x increases, the value of (1/2)^x decreases because raising a fraction between 0 and 1 to a higher power results in a smaller number.Direct Proportionality: Since y is directly proportional to (1/2)^x, as (1/2)^x decreases, y also decreases. Therefore, as x increases, y decreases.Rate of Change: Now we need to determine the rate of change of y as x increases. Because the function is an exponential decay, the rate of decrease of y slows down as x increases. This means that y decreases at a decreasing rate.Correct Graph Statement: Based on the analysis, the correct statement about the graph is that as x increases, y decreases at a decreasing rate. This corresponds to option (D).

Full solution

More problems from Write a quadratic function from its x-intercepts and another point

Related topics