AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
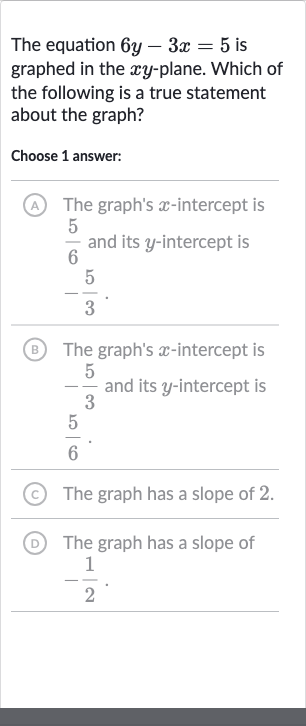
The equation is graphed in the -plane. Which of the following is a true statement about the graph?Choose answer:(A) The graph's -intercept is and its -intercept is .(B) The graph's -intercept is and its -intercept is .(C) The graph has a slope of .D The graph has a slope of .
Full solution
Q. The equation is graphed in the -plane. Which of the following is a true statement about the graph?Choose answer:(A) The graph's -intercept is and its -intercept is .(B) The graph's -intercept is and its -intercept is .(C) The graph has a slope of .D The graph has a slope of .
- Finding the x-intercept: To find the x-intercept, set in the equation and solve for .
- Finding the y-intercept: To find the y-intercept, set in the equation and solve for .
- Finding the slope of the graph: To find the slope of the graph, rewrite the equation in slope-intercept form , where is the slope.The slope of the graph is .
- Check the given options: Option : The graph's -intercept is not and its -intercept is not . Therefore, option is incorrect.Option : The graph's -intercept is and its -intercept is . Therefore, option is correct.Option : The graph does not have a slope of . Therefore, option is incorrect.Option : The graph does not have a slope of . Therefore, option is incorrect.
More problems from Write a quadratic function from its x-intercepts and another point
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
