Full solution
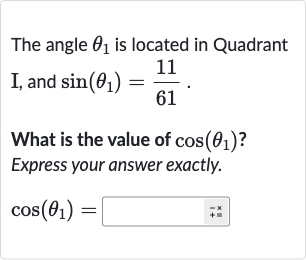
Q. The angle is located in QuadrantI, and .What is the value of ?Express your answer exactly.
- Use Pythagorean Identity: We know that . Use the Pythagorean identity to find the value of .Substitute for in ..
- Substitute : Simplify to find the value of ...
- Simplify to find : Calculate .
.
.
. - Calculate : Since is in Quadrant I, where all trigonometric functions are positive, we take the positive square root of ...