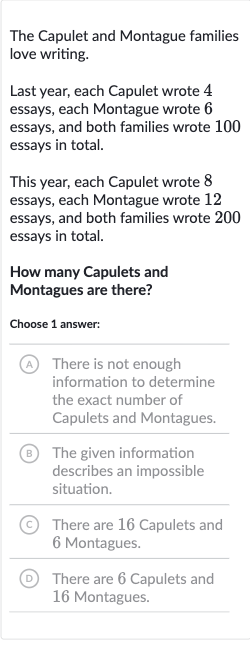
The Capulet and Montague families love writing. Last year, each Capulet wrote 4 essays, each Montague wrote 6 essays, and both families wrote 100 essays in total. This year, each Capulet wrote 8 essays, each Montague wrote 12 essays, and both families wrote 200 essays in total. How many Capulets and Montagues are there? Choose 1 answer: (A) There is not enough information to determine the exact number of Capulets and Montagues. (B) The given information describes an impossible situation. (C) There are 16 Capulets and 6 Montagues. D There are 6 Capulets and 16 Montagues.

Question

Bytelearn · Accepted Answer

Equation 1: Let's denote the number of Capulets as C and the number of Montagues as M. From the first year's information, we can write the following equation based on the total number of essays written: 4C + 6M = 100Equation 2: Similarly, from the second year's information, we can write another equation: 8C + 12M = 200Equation Comparison: We notice that the second equation is exactly double the first equation. This means that if we divide the second equation by 2, we should get the first equation: (8C + 12M) / 2 = 200 / 2 4C + 6M = 100 This confirms that the second equation does not provide new information; it is simply a scaled version of the first equation.Insufficient Information: Since the second equation does not provide new information, we only have one equation with two variables (C and M). This means we cannot solve for the exact values of C and M with the information given. Therefore, the answer is: (A) There is not enough information to determine the exact number of Capulets and Montagues.

Full solution

More problems from Solve a system of equations using any method: word problems

Related topics