Full solution
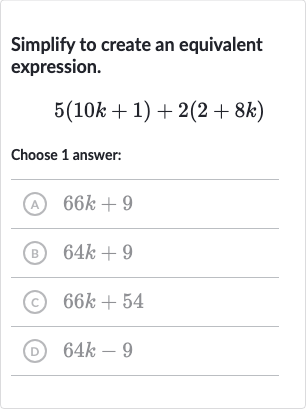
Q. Simplify to create an equivalent expression.Choose answer:(A) (B) (C) (D)
- Distribute : First, distribute the into the terms inside the first set of parentheses: . This gives us .
- Distribute : Next, distribute the into the terms inside the second set of parentheses: . This gives us .
- Combine like terms: Now, combine like terms from both distributed results: + . This gives us .
- Simplified expression: The simplified expression is , which corresponds to option .
More problems from Simplify variable expressions involving like terms and the distributive property
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help