AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
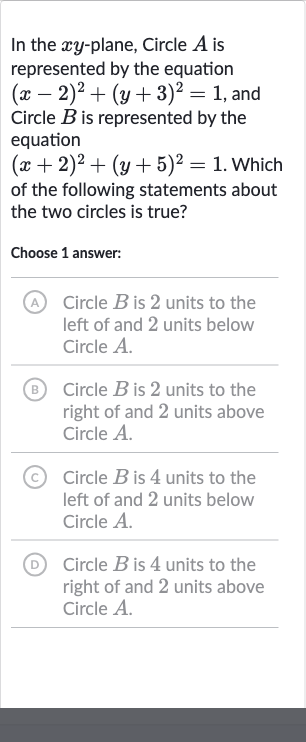
In the -plane, Circle is represented by the equation , and Circle is represented by the equation. Which of the following statements about the two circles is true?Choose answer:(A) Circle is units to the left of and units below Circle .(B) Circle is units to the right of and units above Circle .(C) Circle is units to the left of and units below Circle .(D) Circle is units to the right of and units above Circle .
Full solution
Q. In the -plane, Circle is represented by the equation , and Circle is represented by the equation. Which of the following statements about the two circles is true?Choose answer:(A) Circle is units to the left of and units below Circle .(B) Circle is units to the right of and units above Circle .(C) Circle is units to the left of and units below Circle .(D) Circle is units to the right of and units above Circle .
- Circle A Equation: Circle A is represented by the equation . The center of Circle A is at the point .
- Circle B Equation: Circle B is represented by the equation . The center of Circle B is at the point .
- Comparison of Centers: To compare the positions of Circle and Circle , we need to look at the differences in the -coordinates and -coordinates of their centers.
- Difference in X-coordinates: The difference in the x-coordinates of the centers of Circle A and Circle B is . This means Circle B is units to the left of Circle A.
- Difference in Y-coordinates: The difference in the y-coordinates of the centers of Circle A and Circle B is . This means Circle B is units below Circle A.
- Position Comparison: Based on the differences in the coordinates of the centers, the correct statement about the positions of Circle A and Circle B is that Circle B is units to the left of and units below Circle A.
More problems from Write a quadratic function from its x-intercepts and another point
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
