Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

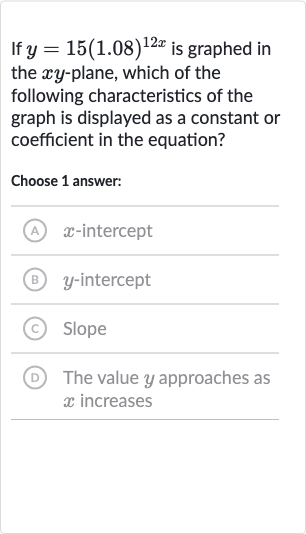
If y=15(1.08)^(12 x) is graphed in the xy-plane, which of the following characteristics of the graph is displayed as a constant or coefficient in the equation? Choose 1 answer: A ) x-intercept (B) y-intercept (C) Slope (D) The value y approaches as x increases

If y=15(1.08)12x y=15(1.08)^{12 x} is graphed in the xy x y -plane, which of the following characteristics of the graph is displayed as a constant or coefficient in the equation?\newlineChoose 11 answer:\newline(A) x x -intercept\newline(B) y y -intercept\newline(C) Slope\newline(D) The value y y approaches as x x increases

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Find trigonometric ratios using a Pythagorean or reciprocal identityright chevron icon

Full solution

Q. If y=15(1.08)12x y=15(1.08)^{12 x} is graphed in the xy x y -plane, which of the following characteristics of the graph is displayed as a constant or coefficient in the equation?\newlineChoose 11 answer:\newline(A) x x -intercept\newline(B) y y -intercept\newline(C) Slope\newline(D) The value y y approaches as x x increases
  1. Identifying Constants and Coefficients: We need to identify which characteristic of the graph is represented by a constant or coefficient in the given equation y=15(1.08)12xy=15(1.08)^{12x}. Let's analyze the equation.
  2. Meaning of Constant 'a': The equation is in the form y=a(b)cxy=a(b)^{cx}, where aa, bb, and cc are constants. In this case, a=15a=15, b=1.08b=1.08, and c=12c=12. We need to determine what each of these constants represents in the graph.
  3. Meaning of Constant 'b': The constant 'aa' (which is 1515 in this equation) represents the initial value of yy when xx is 00. This is because anything raised to the power of 00 is 11, so y=a(1)y=a(1) when x=0x=0, which simplifies to y=ay=a. Therefore, the constant 'aa' represents the yy-intercept of the graph.
  4. Meaning of Constant 'c': The constant 'bb' (which is 1.081.08 in this equation) represents the base of the exponential function. It affects the rate of growth or decay of the graph but does not directly represent a characteristic like the yy-intercept, xx-intercept, or slope.
  5. X-Intercept and Exponential Functions: The constant cc (which is 1212 in this equation) is the coefficient of xx in the exponent. It affects the rate at which the function grows or decays, but like bb, it does not directly represent a characteristic like the y-intercept, x-intercept, or slope.
  6. Slope of an Exponential Function: The xx-intercept is the value of xx when y=0y=0. Since an exponential function like this one never touches the xx-axis (assuming b>1, which it is in this case), the xx-intercept is not represented by any constant or coefficient in the equation.
  7. Horizontal Asymptote and Exponential Growth: The slope of an exponential function is not constant; it changes at every point on the graph. Therefore, it is not represented by a single constant or coefficient in the equation.
  8. Horizontal Asymptote and Exponential Growth: The slope of an exponential function is not constant; it changes at every point on the graph. Therefore, it is not represented by a single constant or coefficient in the equation.The value yy approaches as xx increases is known as the horizontal asymptote. For exponential growth functions where b>1, yy approaches infinity as xx increases, and this behavior is not represented by a specific constant or coefficient in the equation.

More problems from Find trigonometric ratios using a Pythagorean or reciprocal identity

Question
If cos⁡(θ)=817 \cos(\theta)=\frac{8}{17} and 0∘<θ<90∘ 0^\circ<\theta<90^\circ , what is sec⁡(θ) \sec(\theta) ? Write your answer in simplified, rationalized form. sec⁡(θ)= \sec(\theta)= ______
Get tutor helpright-arrow

Posted 5 months ago

Question
cos⁡(x)=−0.3\cos(x) = -0.3. What is sin⁡(90∘−x)\sin(90^\circ - x)? ____
Get tutor helpright-arrow

Posted 8 months ago

Question
If csc⁡(θ)=178 \csc(\theta) = \frac{17}{8} and 0∘<θ<90∘ 0^\circ < \theta < 90^\circ , what is tan⁡(θ) \tan(\theta) ?\newlineWrite your answer in simplified, rationalized form.\newlinetan⁡(θ)= \tan(\theta) = ______\newline
Get tutor helpright-arrow

Posted 8 months ago

Question
Is the sine function even or odd?\newlineChoices:\newline[A]even\text{[A]even}\newline[B]odd\text{[B]odd}
Get tutor helpright-arrow

Posted 8 months ago

Question
Write the sentence as an equation. \newline8989 is equal to the quotient of yy and 1717 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 7 months ago

Question
Write the sentence as an equation. \newline4545 is equal to the quotient of zz and 55 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for hh. \newline h2+24h=0h^2 + 24h = 0 \newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas. \newline h=h = _____
Get tutor helpright-arrow

Posted 9 months ago

Question
h2−4h=0h^2 - 4h = 0
Get tutor helpright-arrow

Posted 7 months ago

Question
Combine the like terms to create an equivalent expression:\newline−k−(−8k)=□-k-(-8k)=\square
Get tutor helpright-arrow

Posted 10 months ago

Question
Which of the following degree measures is equal to 5π 5 \pi radians?\newline(The number of degrees of arc in a circle is 360360 . The number of radians of arc in a circle is 2π 2 \pi .)\newlineChoose 11 answer:\newline(A) 144∘ 144^{\circ} \newline(B) 900∘ 900^{\circ} \newline(C) 1,080∘ 1,080^{\circ} \newline(D) 1,800∘ 1,800^{\circ}
Get tutor helpright-arrow

Posted 7 months ago

View all (24)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant