Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

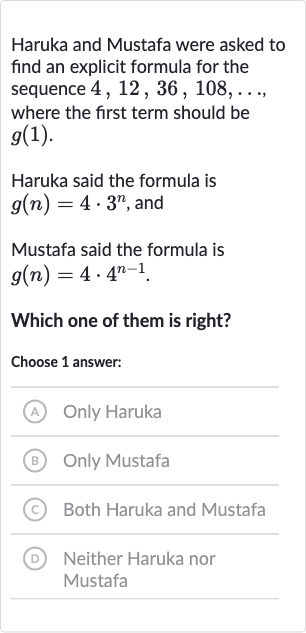
Haruka and Mustafa were asked to find an explicit formula for the sequence 4,12,36,108,dots, where the first term should be g(1). Haruka said the formula is g(n)=4*3^(n), and Mustafa said the formula is g(n)=4*4^(n-1). Which one of them is right? Choose 1 answer: (A) Only Haruka (B) Only Mustafa (c) Both Haruka and Mustafa (D) Neither Haruka nor Mustafa

Haruka and Mustafa were asked to find an explicit formula for the sequence \newline4,12,36,108,…4,12,36,108,\dots, where the first term should be \newlineg(1)g(1).\newlineHaruka said the formula is \newlineg(n)=4⋅3ng(n)=4\cdot3^{n}, and\newlineMustafa said the formula is \newlineg(n)=4⋅4n−1g(n)=4\cdot4^{n-1}.\newlineWhich one of them is right?\newlineChoose 11 answer:\newline(A) Only Haruka\newline(B) Only Mustafa\newline(C) Both Haruka and Mustafa\newline(D) Neither Haruka nor Mustafa

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Write variable expressions for geometric sequencesright chevron icon

Full solution

Q. Haruka and Mustafa were asked to find an explicit formula for the sequence \newline4,12,36,108,…4,12,36,108,\dots, where the first term should be \newlineg(1)g(1).\newlineHaruka said the formula is \newlineg(n)=4⋅3ng(n)=4\cdot3^{n}, and\newlineMustafa said the formula is \newlineg(n)=4⋅4n−1g(n)=4\cdot4^{n-1}.\newlineWhich one of them is right?\newlineChoose 11 answer:\newline(A) Only Haruka\newline(B) Only Mustafa\newline(C) Both Haruka and Mustafa\newline(D) Neither Haruka nor Mustafa
  1. Identifying the Pattern: We have the sequence: 4,12,36,108,…4, 12, 36, 108, \ldots\newlineTo determine which formula is correct, we need to identify the pattern of the sequence.
  2. Checking Haruka's Formula: The sequence appears to be geometric because each term is multiplied by the same factor to get the next term.\newlineTo find this common ratio rr, we divide the second term by the first term: r=124=3r = \frac{12}{4} = 3.
  3. Checking Mustafa's Formula: Now, let's check Haruka's formula: g(n)=4⋅3ng(n) = 4 \cdot 3^n.\newlineFor n=1n = 1, g(1)=4⋅31=4⋅3=12g(1) = 4 \cdot 3^1 = 4 \cdot 3 = 12, which does not match the first term of the sequence.\newlineTherefore, Haruka's formula is incorrect.
  4. Checking Mustafa's Formula: Now, let's check Haruka's formula: g(n)=4⋅3ng(n) = 4 \cdot 3^n. For n=1n = 1, g(1)=4⋅31=4⋅3=12g(1) = 4 \cdot 3^1 = 4 \cdot 3 = 12, which does not match the first term of the sequence. Therefore, Haruka's formula is incorrect.Let's check Mustafa's formula: g(n)=4⋅4(n−1)g(n) = 4 \cdot 4^{(n-1)}. For n=1n = 1, g(1)=4⋅4(1−1)=4⋅40=4⋅1=4g(1) = 4 \cdot 4^{(1-1)} = 4 \cdot 4^0 = 4 \cdot 1 = 4, which matches the first term of the sequence. For n=2n = 2, g(2)=4⋅4(2−1)=4⋅41=4⋅4=16g(2) = 4 \cdot 4^{(2-1)} = 4 \cdot 4^1 = 4 \cdot 4 = 16, which does not match the second term of the sequence. Therefore, Mustafa's formula is also incorrect.

More problems from Write variable expressions for geometric sequences

Question
Find the sum of the finite arithmetic series. ∑n=110(7n+4)\sum_{n=1}^{10} (7n+4)\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Type the missing number in this sequence: `55,\59,\63,\text{_____},\71,\75,\79`
Get tutor helpright-arrow

Posted 5 months ago

Question
Type the missing number in this sequence: `2, 4 8`, ______,`32`
Get tutor helpright-arrow

Posted 5 months ago

Question
What kind of sequence is this? 2,10,50,250,…2, 10, 50, 250, \ldots Choices:Choices:\newline[A]arithmetic\text{[A]arithmetic}\newline[B]geometric\text{[B]geometric}\newline[C]both\text{[C]both}\newline[D]neither\text{[D]neither}
Get tutor helpright-arrow

Posted 5 months ago

Question
What is the missing number in this pattern? 1,4,9,16,25,36,49,64,81,____1, 4, 9, 16, 25, 36, 49, 64, 81, \_\_\_\_
Get tutor helpright-arrow

Posted 7 months ago

Question
Classify the series. ∑n=012(n+2)3 \sum_{n = 0}^{12} (n + 2)^3 \newlineChoices:\newline[A]arithmetic\text{[A]arithmetic}\newline[B]geometric\text{[B]geometric}\newline[C]both\text{[C]both}\newline[D]neither\text{[D]neither}
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the first three partial sums of the series.\newline1+6+11+16+21+26+⋯1 + 6 + 11 + 16 + 21 + 26 + \cdots\newlineWrite your answers as integers or fractions in simplest form.\newlineS1=S_1 = ____\newlineS2=S_2 = ____\newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find the third partial sum of the series. \newline3+9+15+21+27+33+⋯3 + 9 + 15 + 21 + 27 + 33 + \cdots\newlineWrite your answer as an integer or a fraction in simplest form. \newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find the first three partial sums of the series. \newline1+7+13+19+25+31+⋯1 + 7 + 13 + 19 + 25 + 31 + \cdots\newlineWrite your answers as integers or fractions in simplest form. \newlineS1=S_1 = ____\newlineS2=S_2 = ____\newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 9 months ago

Question
Does the infinite geometric series converge or diverge?\newline1+34+916+2764+⋯1 + \frac{3}{4} + \frac{9}{16} + \frac{27}{64} + \cdots\newlineChoices:\newline[A]converge\text{[A]converge}\newline[B]diverge\text{[B]diverge}
Get tutor helpright-arrow

Posted 5 months ago

View all (52)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant