AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
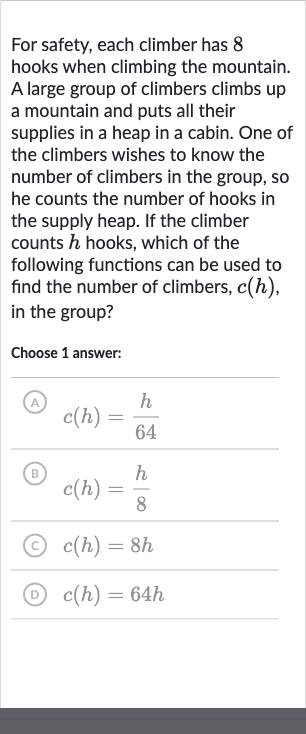
For safety, each climber has hooks when climbing the mountain. A large group of climbers climbs up a mountain and puts all their supplies in a heap in a cabin. One of the climbers wishes to know the number of climbers in the group, so he counts the number of hooks in the supply heap. If the climber counts hooks, which of the following functions can be used to find the number of climbers, , in the group?Choose answer:(A) (B) (C) (D)
Full solution
Q. For safety, each climber has hooks when climbing the mountain. A large group of climbers climbs up a mountain and puts all their supplies in a heap in a cabin. One of the climbers wishes to know the number of climbers in the group, so he counts the number of hooks in the supply heap. If the climber counts hooks, which of the following functions can be used to find the number of climbers, , in the group?Choose answer:(A) (B) (C) (D)
- Calculate Total Climbers: Each climber has hooks. To find the total number of climbers based on the total number of hooks, we need to divide the total number of hooks by the number of hooks per climber.
- Define Function: The function that represents the number of climbers, , based on the number of hooks, , is . This is because if you divide the total number of hooks by the number of hooks each climber has, you get the total number of climbers.
