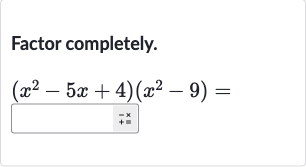AI tutor
Full solution
Q. Factor completely.
- Factor the quadratic expression: Factor the quadratic expression .We look for two numbers that multiply to and add up to . These numbers are and .So, we can factor as .
- Factor the difference of squares: Factor the difference of squares .We recognize that is a perfect square, and the expression is in the form , which factors into .Here, is and is , so factors into .
- Combine the factors: Combine the factors from Step and Step to write the complete factorization.The complete factorization of the given expression is .
More problems from Factor sums and differences of cubes
QuestionGet tutor help
QuestionGet tutor help