AI tutor
Full solution
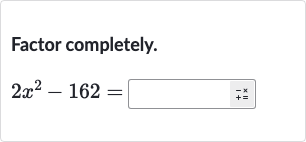
Q. Factor completely.
- Identify common factor: Identify if there is a common factor in the terms and .Both terms are even, so they have a common factor of .Factor out the :
- Factor out the common factor: Recognize that is a difference of squares.So,
- Recognize difference of squares: Use the difference of squares formula to factor .The formula is .
- Use difference of squares formula: Combine the factored form of with the common factor that was factored out in Step .