AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
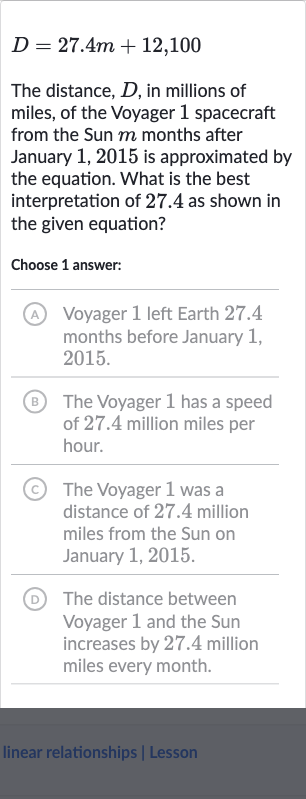
The distance, , in millions of miles, of the Voyager spacecraft from the Sun months after January , is approximated by the equation. What is the best interpretation of . as shown in the given equation?Choose answer:(A) Voyager left Earth . months before January , .(B) The Voyager has a speed of . million miles per hour.(C) The Voyager was a distance of . million miles from the Sun on January , .(D) The distance between Voyager and the Sun increases by . million miles every month.linear relationships | Lesson
Full solution
Q. The distance, , in millions of miles, of the Voyager spacecraft from the Sun months after January , is approximated by the equation. What is the best interpretation of . as shown in the given equation?Choose answer:(A) Voyager left Earth . months before January , .(B) The Voyager has a speed of . million miles per hour.(C) The Voyager was a distance of . million miles from the Sun on January , .(D) The distance between Voyager and the Sun increases by . million miles every month.linear relationships | Lesson
- Understand Coefficient Role: We are given the equation , where is the distance in millions of miles and is the number of months after January , . To interpret the coefficient , we need to understand its role in the equation.
- Linear Equation Interpretation: The equation is linear, which means that for every increase in , the distance increases by the coefficient of . In this case, the coefficient is , which means that for every month, the distance increases by million miles.
- Option Evaluation A: Now, let's evaluate the options given:(A) This option suggests that Voyager left Earth months before January , . This interpretation is not supported by the equation, as the coefficient is multiplied by the number of months after January , , not before.
- Option Evaluation B: (B) This option suggests that Voyager has a speed of million miles per hour. However, the equation does not mention hours, and the coefficient is associated with the number of months, not hours. Therefore, this interpretation is incorrect.
- Option Evaluation C: (C) This option suggests that Voyager was a distance of million miles from the Sun on January , . This interpretation is incorrect because the constant term in the equation () represents the initial distance from the Sun on January , , not the coefficient .
- Option Evaluation D: (D) This option suggests that the distance between Voyager and the Sun increases by million miles every month. This interpretation is consistent with the role of the coefficient in the linear equation, which indicates the rate of change of the distance with respect to time (months).
