Resources

Testimonials

Plans

Resources

Testimonials

Plans

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

$Combine like terms to simplify the expression: Enter any coefficients as simplified proper or improper fractions or integers. (2)/(5)k-(3)/(5)+(1)/(10)k$

Combine like terms to simplify the expression: $\newline$ Enter any coefficients as simplified proper or improper fractions or integers. $\newline$ $(\frac{2}{5})k-(\frac{3}{5})+(\frac{1}{10})k$

View step-by-step help

Home

Math Problems

Algebra 1

Simplify variable expressions involving like terms and the distributive property

Full solution

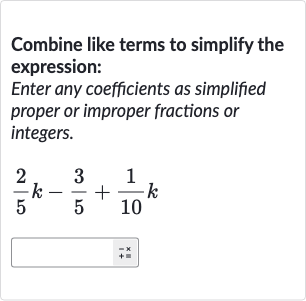
Q. Combine like terms to simplify the expression:

\newline

Enter any coefficients as simplified proper or improper fractions or integers.

\newline

(\frac{2}{5})k-(\frac{3}{5})+(\frac{1}{10})k

Combine like terms: Combine like terms by adding the coefficients of $k$ .
$\frac{2}{5}k + \frac{1}{10}k - \frac{3}{5}$
To combine the terms with $k$ , find a common denominator for the fractions. The common denominator for $5$ and $10$ is $10$ .
Convert $(\frac{2}{5})k$ to a fraction: Convert $(\frac{2}{5})k$ to a fraction with a denominator of $10$ . $(\frac{2}{5})k = (\frac{2 \times 2}{5 \times 2})k = (\frac{4}{10})k$
Add the coefficients of $k$ : Now add the coefficients of $k$ . $\newline$ $(\frac{4}{10})k + (\frac{1}{10})k = (\frac{4}{10} + \frac{1}{10})k = (\frac{5}{10})k$ $\newline$ Simplify the fraction $(\frac{5}{10})$ to $(\frac{1}{2})$ . $\newline$ $(\frac{5}{10})k = (\frac{1}{2})k$
Simplify the fraction: The constant term remains unchanged since there are no like terms to combine it with. $\newline$ So the expression is now $(\frac{1}{2})k - (\frac{3}{5})$ .