Resources

Testimonials

Plans

Resources

Testimonials

Plans

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

$Combine like terms to create an equivalent expression. Enter any coefficients as simplified proper or improper fractions or integers. -(2)/(3)p+(1)/(5)-1+(5)/(6)p$

- $\frac{2}{3}p + \frac{1}{5} - 1 + \frac{5}{6}p$

View step-by-step help

Home

Math Problems

Algebra 1

Simplify variable expressions involving like terms and the distributive property

Full solution

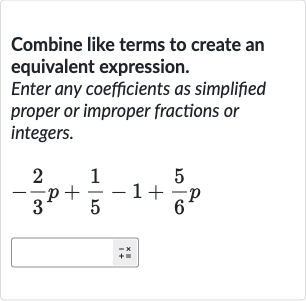
Q. -

\frac{2}{3}p + \frac{1}{5} - 1 + \frac{5}{6}p

Identify like terms: Identify like terms in the expression. $\newline$ The like terms in the expression are the terms that contain the variable ' extit{p}'. In this case, the like terms are $-(\frac{2}{3})p$ and $(\frac{5}{6})p$ . The constants $(\frac{1}{5})$ and $-1$ are also like terms.
Combine like terms with 'p': Combine the like terms that contain the variable 'p'. $\newline$ To combine $-(\frac{2}{3})p$ and $(\frac{5}{6})p$ , find a common denominator, which is $6$ in this case, and then add the fractions. $\newline$ $-(\frac{2}{3})p = -(\frac{4}{6})p$ (by multiplying both the numerator and the denominator by $2$ ) $\newline$ $(\frac{5}{6})p$ remains the same. $\newline$ Now, add $-(\frac{4}{6})p$ and $(\frac{5}{6})p$ : $\newline$ $-(\frac{4}{6})p + (\frac{5}{6})p = (\frac{5}{6} - \frac{4}{6})p = (\frac{1}{6})p$
Combine constant terms: Combine the constant terms $(\frac{1}{5})$ and $-1$ . $\newline$ To combine $(\frac{1}{5})$ and $-1$ , convert $-1$ into a fraction with a denominator of $5$ : $\newline$ $-1 = -(\frac{5}{5})$ $\newline$ Now, add $(\frac{1}{5})$ and $-(\frac{5}{5})$ : $\newline$ $(\frac{1}{5}) + -(\frac{5}{5}) = (\frac{1}{5} - \frac{5}{5}) = -(\frac{4}{5})$
Write final simplified expression: Write the final simplified expression. $\newline$ Combine the results from Step $2$ and Step $3$ to write the final expression: $\newline$ $(\frac{1}{6})p - (\frac{4}{5})$