Resources

Testimonials

Plans

Resources

Testimonials

Plans

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

$Combine like terms to create an equivalent expression. Enter any coefficients as simplified proper or improper fractions or integers. (1)/(7)-3((3)/(7)n-(2)/(7))$

Combine like terms to create an equivalent expression. $\newline$ Enter any coefficients as simplified proper or improper fractions or integers. $\newline$ $\frac{1}{7}-3\left(\frac{3}{7}n-\frac{2}{7}\right)$

View step-by-step help

Home

Math Problems

Algebra 1

Simplify variable expressions involving like terms and the distributive property

Full solution

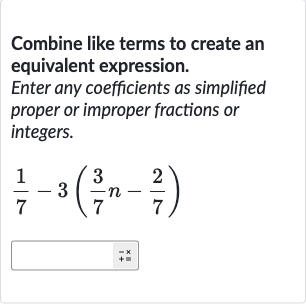
Q. Combine like terms to create an equivalent expression.

\newline

Enter any coefficients as simplified proper or improper fractions or integers.

\newline

\frac{1}{7}-3\left(\frac{3}{7}n-\frac{2}{7}\right)

Identify and group like terms: Identify and group like terms in the expression $(\frac{1}{7}) - 3\left((\frac{3}{7})n - (\frac{2}{7})\right)$ . $\newline$ The like terms to be combined are the terms involving 'n'. $\newline$ Expression: $(\frac{1}{7}) - 3 \times (\frac{3}{7})n + 3 \times (\frac{2}{7})$
Distribute the $-3$ : Distribute the $-3$ across the terms inside the parentheses. $\newline$ $(\frac{1}{7}) - 3 \times (\frac{3}{7})n + 3 \times (\frac{2}{7}) = (\frac{1}{7}) - (\frac{9}{7})n + (\frac{6}{7})$
Combine constant terms: Combine the constant terms $(\frac{1}{7})$ and $(\frac{6}{7})$ . $\newline$ $(\frac{1}{7}) + (\frac{6}{7}) = (\frac{7}{7})$
Simplify constant term: Simplify the constant term $(\frac{7}{7})$ . $\newline$ $(\frac{7}{7}) = 1$
Write final expression: Write the final expression after combining like terms. $\newline$ Final expression: $1 - \left(\frac{9}{7}\right)n$