Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

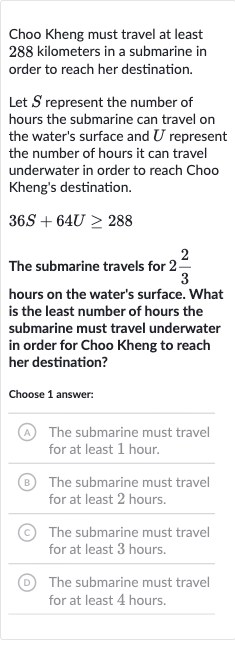
Choo Kheng must travel at least 288 kilometers in a submarine in order to reach her destination. Let S represent the number of hours the submarine can travel on the water's surface and U represent the number of hours it can travel underwater in order to reach Choo Kheng's destination. 36 S+64 U >= 288 The submarine travels for 2(2)/(3) hours on the water's surface. What is the least number of hours the submarine must travel underwater in order for Choo Kheng to reach her destination? Choose 1 answer: (A) The submarine must travel for at least 1 hour. (B) The submarine must travel for at least 2 hours. (C) The submarine must travel for at least 3 hours. D The submarine must travel for at least 4 hours.

Choo Kheng must travel at least 288288 kilometers in a submarine in order to reach her destination.\newlineLet S S represent the number of hours the submarine can travel on the water's surface and U U represent the number of hours it can travel underwater in order to reach Choo Kheng's destination.\newline36S+64U≥288 36 S+64 U \geq 288 \newlineThe submarine travels for 223 2 \frac{2}{3} hours on the water's surface. What is the least number of hours the submarine must travel underwater in order for Choo Kheng to reach her destination?\newlineChoose 11 answer:\newline(A) The submarine must travel for at least 11 hour.\newline(B) The submarine must travel for at least 22 hours.\newline(C) The submarine must travel for at least 33 hours.\newline(D) The submarine must travel for at least 44 hours.

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Interpret parts of quadratic expressions: word problemsright chevron icon

Full solution

Q. Choo Kheng must travel at least 288288 kilometers in a submarine in order to reach her destination.\newlineLet S S represent the number of hours the submarine can travel on the water's surface and U U represent the number of hours it can travel underwater in order to reach Choo Kheng's destination.\newline36S+64U≥288 36 S+64 U \geq 288 \newlineThe submarine travels for 223 2 \frac{2}{3} hours on the water's surface. What is the least number of hours the submarine must travel underwater in order for Choo Kheng to reach her destination?\newlineChoose 11 answer:\newline(A) The submarine must travel for at least 11 hour.\newline(B) The submarine must travel for at least 22 hours.\newline(C) The submarine must travel for at least 33 hours.\newline(D) The submarine must travel for at least 44 hours.
  1. Understand the problem: Understand the problem.\newlineWe are given the equation 36S+64U≥28836S + 64U \geq 288, where SS is the number of hours the submarine travels on the water's surface and UU is the number of hours it travels underwater. We need to find the minimum value of UU when SS is given as 2(23)2\left(\frac{2}{3}\right) hours.
  2. Substitute and simplify: Substitute the given value of SS into the equation.\newlineSS is given as 2(23)2\left(\frac{2}{3}\right) hours, which is equal to 83\frac{8}{3} hours. We substitute this value into the equation to find UU.\newline36(83)+64U≥28836\left(\frac{8}{3}\right) + 64U \geq 288
  3. Perform multiplication: Perform the multiplication to simplify the equation.\newline36×(83)=9636 \times \left(\frac{8}{3}\right) = 96\newlineSo, the equation becomes:\newline96+64U≥28896 + 64U \geq 288
  4. Perform subtraction: Subtract 9696 from both sides of the inequality to solve for UU. \newline64U≥288−9664U \geq 288 - 96\newline64U≥19264U \geq 192
  5. Divide to find minimum: Divide both sides of the inequality by 6464 to find the minimum value of UU.\newlineU≥19264U \geq \frac{192}{64}\newlineU≥3U \geq 3

More problems from Interpret parts of quadratic expressions: word problems

Question
Solve by completing the square.\newlinem2−10m−29=0m^2 - 10m - 29 = 0\newlineWrite your answers as integers, proper or improper fractions in simplest form, or decimals rounded to the nearest hundredth.\newline`m` = ____ or `m` = _____
Get tutor helpright-arrow

Posted 8 months ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 5 5 units up of f(x)=x2 f(x)=x^2 .\newlineWrite your answer in the form a(x–h)2+k a(x–h)^2+k , where a a , h h , and k k are integers.\newlineg(x)= g(x)= ____
Get tutor helpright-arrow

Posted 7 months ago

Question
What is the range of this quadratic function?\newliney=x2−4x+4y = x^2 - 4x + 4\newlineChoices:\newline{y∣y≥2}\left\{y \mid y \geq 2\right\}\newline{y∣y≤0}\left\{y \mid y \leq 0\right\}\newline{y∣y≥0}\left\{y \mid y \geq 0\right\}\newlineall real numbers\text{all real numbers}
Get tutor helpright-arrow

Posted 5 months ago

Question
Write the equation of the parabola that passes through the points (1,0)(1,0), (2,0)(2,0), and (3,–16)(3,\text{–}16). Write your answer in the form y=a(x–p)(x–q)y = a(x – p)(x – q), where aa, pp, and qq are integers, decimals, or simplified fractions.\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Complete the square. Fill in the number that makes the polynomial a perfect-square quadratic.\newlinef2+8f+_____f^2 + 8f + \_\_\_\_\_
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve for h h .\newlineh2+39h=0 h^2 + 39h = 0 \newline\newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas.\newlineh= h = ____\newline
Get tutor helpright-arrow

Posted 5 months ago

Question
Write a quadratic function with zeros −9-9 and −7-7.\newlineWrite your answer using the variable xx and in standard form with a leading coefficient of 11.\newlinef(x)=_____f(x) = \_\_\_\_\_
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the equation of the axis of symmetry for the parabola y=x2y = x^2. \newlineSimplify any numbers and write them as proper fractions, improper fractions, or integers.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 4 months ago

Question
Find g(x)g(x), where g(x)g(x) is the translation 88 units up of f(x)=x2f(x) = x^2.\newlineWrite your answer in the form a(x–h)2+ka(x – h)^2 + k, where aa, hh, and kk are integers.\newlineg(x)=g(x) = ______\newline
Get tutor helpright-arrow

Posted 4 months ago

Question
Solve for xx.\newlinex2=1x^2 = 1\newline\newlineWrite your answer in simplified, rationalized form.\newlinex=x = ______ or x=x = ______\newline
Get tutor helpright-arrow

Posted 5 months ago

View all (145)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant