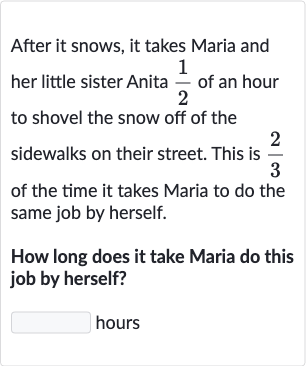
After it snows, it takes Maria and her little sister Anita (1)/(2) of an hour to shovel the snow off of the sidewalks on their street. This is (2)/(3) of the time it takes Maria to do the same job by herself. How long does it take Maria do this job by herself? hours

Question

After it snows, it takes Maria and her little sister Anita  (1)/(2) of an hour to shovel the snow off of the sidewalks on their street. This is  (2)/(3) of the time it takes Maria to do the same job by herself. How long does it take Maria do this job by herself? hours

Bytelearn · Accepted Answer

Set Up Equation: Let's denote the time it takes Maria to shovel the snow by herself as T hours. According to the problem, Maria and her sister together take 1/2 hour to complete the task, and this time is 2/3 of the time Maria would take by herself. We can set up the following equation to represent this relationship: (1/2) hour = (2/3) * TIsolate T: Now we need to solve for T. To do this, we can divide both sides of the equation by (2/3) to isolate T on one side: T = (1/2) / (2/3)Divide Fractions: To divide fractions, we multiply by the reciprocal of the divisor. The reciprocal of (2/3) is (3/2), so we multiply (1/2) by (3/2): T = (1/2) * (3/2)Multiply Reciprocals: Multiplying the numerators and denominators separately, we get: T = (1 * 3) / (2 * 2) T = 3 / 4Final Answer: So, it takes Maria 3/4 of an hour to shovel the snow off of the sidewalks on their street by herself.

After it snows, it takes Maria and her little sister Anita $\frac{1}{2}$ of an hour to shovel the snow off of the sidewalks on their street. This is $\frac{2}{3}$ of the time it takes Maria to do the same job by herself. $\newline$ How long does it take Maria do this job by herself? $\newline$ hours

Full solution

More problems from GCF and LCM: word problems

Related topics