Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

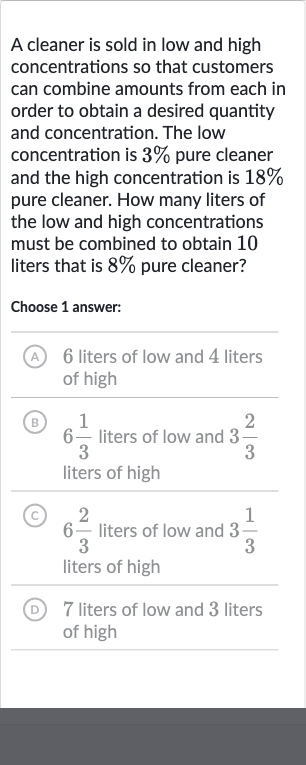
A cleaner is sold in low and high concentrations so that customers can combine amounts from each in order to obtain a desired quantity and concentration. The low concentration is 3% pure cleaner and the high concentration is 18% pure cleaner. How many liters of the low and high concentrations must be combined to obtain 10 liters that is 8% pure cleaner? Choose 1 answer: A 6 liters of low and 4 liters of high (B) 6(1)/(3) liters of low and 3(2)/(3) liters of high (C) 6(2)/(3) liters of low and 3(1)/(3) liters of high (D) 7 liters of low and 3 liters of high

A cleaner is sold in low and high concentrations so that customers can combine amounts from each in order to obtain a desired quantity and concentration. The low concentration is 3% 3 \% pure cleaner and the high concentration is 18% 18 \% pure cleaner. How many liters of the low and high concentrations must be combined to obtain 1010 liters that is 8% 8 \% pure cleaner?\newlineChoose 11 answer:\newline(A) 66 liters of low and 44 liters of high\newline(B) 613 6 \frac{1}{3} liters of low and 323 3 \frac{2}{3} liters of high\newline(C) 623 6 \frac{2}{3} liters of low and 313 3 \frac{1}{3} liters of high\newline(D) 77 liters of low and 33 liters of high

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Interpret parts of quadratic expressions: word problemsright chevron icon

Full solution

Q. A cleaner is sold in low and high concentrations so that customers can combine amounts from each in order to obtain a desired quantity and concentration. The low concentration is 3% 3 \% pure cleaner and the high concentration is 18% 18 \% pure cleaner. How many liters of the low and high concentrations must be combined to obtain 1010 liters that is 8% 8 \% pure cleaner?\newlineChoose 11 answer:\newline(A) 66 liters of low and 44 liters of high\newline(B) 613 6 \frac{1}{3} liters of low and 323 3 \frac{2}{3} liters of high\newline(C) 623 6 \frac{2}{3} liters of low and 313 3 \frac{1}{3} liters of high\newline(D) 77 liters of low and 33 liters of high
  1. Define Variables: Let's denote the amount of low concentration cleaner as LL liters and the amount of high concentration cleaner as HH liters. We know that the total amount of cleaner we want is 1010 liters, so we can write the first equation:\newlineL+H=10L + H = 10
  2. Set Up Equations: We also know that the final mixture should be 8%8\% pure cleaner. We can write a second equation representing the total amount of pure cleaner in the final mixture:\newline0.03L+0.18H=0.08×100.03L + 0.18H = 0.08 \times 10
  3. Solve System: Now we have a system of two equations with two variables:\newline11) L+H=10L + H = 10\newline22) 0.03L+0.18H=0.80.03L + 0.18H = 0.8\newlineWe can solve this system using substitution or elimination. Let's use the substitution method. From the first equation, we can express LL in terms of HH:\newlineL=10−HL = 10 - H
  4. Substitute and Expand: Substitute L=10−HL = 10 - H into the second equation:\newline0.03(10−H)+0.18H=0.80.03(10 - H) + 0.18H = 0.8\newlineExpand the equation:\newline0.3−0.03H+0.18H=0.80.3 - 0.03H + 0.18H = 0.8\newlineCombine like terms:\newline0.15H=0.50.15H = 0.5
  5. Find High Concentration: Now, solve for HH: \newlineH=0.50.15H = \frac{0.5}{0.15}\newlineH=103H = \frac{10}{3}\newlineH=3(13)H = 3\left(\frac{1}{3}\right) liters
  6. Find Low Concentration: Now that we have HH, we can find LL using the first equation:\newlineL=10−HL = 10 - H\newlineL=10−3(13)L = 10 - 3(\frac{1}{3})\newlineL=10−103L = 10 - \frac{10}{3}\newlineL=303−103L = \frac{30}{3} - \frac{10}{3}\newlineL=203L = \frac{20}{3}\newlineL=6(23)L = 6(\frac{2}{3}) liters
  7. Final Answer: We have found the amounts of low and high concentration cleaners needed:\newlineL = 6(23)6\left(\frac{2}{3}\right) liters of low concentration\newlineH = 3(13)3\left(\frac{1}{3}\right) liters of high concentration\newlineThis corresponds to answer choice (C)(C).

More problems from Interpret parts of quadratic expressions: word problems

Question
Solve by completing the square.\newlinem2−10m−29=0m^2 - 10m - 29 = 0\newlineWrite your answers as integers, proper or improper fractions in simplest form, or decimals rounded to the nearest hundredth.\newline`m` = ____ or `m` = _____
Get tutor helpright-arrow

Posted 8 months ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 5 5 units up of f(x)=x2 f(x)=x^2 .\newlineWrite your answer in the form a(x–h)2+k a(x–h)^2+k , where a a , h h , and k k are integers.\newlineg(x)= g(x)= ____
Get tutor helpright-arrow

Posted 7 months ago

Question
What is the range of this quadratic function?\newliney=x2−4x+4y = x^2 - 4x + 4\newlineChoices:\newline{y∣y≥2}\left\{y \mid y \geq 2\right\}\newline{y∣y≤0}\left\{y \mid y \leq 0\right\}\newline{y∣y≥0}\left\{y \mid y \geq 0\right\}\newlineall real numbers\text{all real numbers}
Get tutor helpright-arrow

Posted 5 months ago

Question
Write the equation of the parabola that passes through the points (1,0)(1,0), (2,0)(2,0), and (3,–16)(3,\text{–}16). Write your answer in the form y=a(x–p)(x–q)y = a(x – p)(x – q), where aa, pp, and qq are integers, decimals, or simplified fractions.\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Complete the square. Fill in the number that makes the polynomial a perfect-square quadratic.\newlinef2+8f+_____f^2 + 8f + \_\_\_\_\_
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve for h h .\newlineh2+39h=0 h^2 + 39h = 0 \newline\newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas.\newlineh= h = ____\newline
Get tutor helpright-arrow

Posted 5 months ago

Question
Write a quadratic function with zeros −9-9 and −7-7.\newlineWrite your answer using the variable xx and in standard form with a leading coefficient of 11.\newlinef(x)=_____f(x) = \_\_\_\_\_
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the equation of the axis of symmetry for the parabola y=x2y = x^2. \newlineSimplify any numbers and write them as proper fractions, improper fractions, or integers.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 4 months ago

Question
Find g(x)g(x), where g(x)g(x) is the translation 88 units up of f(x)=x2f(x) = x^2.\newlineWrite your answer in the form a(x–h)2+ka(x – h)^2 + k, where aa, hh, and kk are integers.\newlineg(x)=g(x) = ______\newline
Get tutor helpright-arrow

Posted 4 months ago

Question
Solve for xx.\newlinex2=1x^2 = 1\newline\newlineWrite your answer in simplified, rationalized form.\newlinex=x = ______ or x=x = ______\newline
Get tutor helpright-arrow

Posted 5 months ago

View all (97)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant