A circle in the $x y$ -plane has the equation $\newline$ $x^{2}+y^{2}-10 x+32 y+272=0 \text {. }$ $\newline$ Which of the following best describes the location of the center of the circle and the length of its radius? $\newline$ Choose $1$ answer: $\newline$ (A) Center: $(10,-32)$ $\newline$ Radius: $4 \sqrt{17}$ $\newline$ (B) Center: $(-10,32)$ $\newline$ Radius: $4 \sqrt{17}$ $\newline$ (C) Center: $(-5,16)$ $\newline$ Radius: $3$ $\newline$ (D) Center: $(5,-16)$ $\newline$ Radius: $3$

View step-by-step help

Home

Math Problems

Geometry

Find properties of circles from equations in general form

Full solution

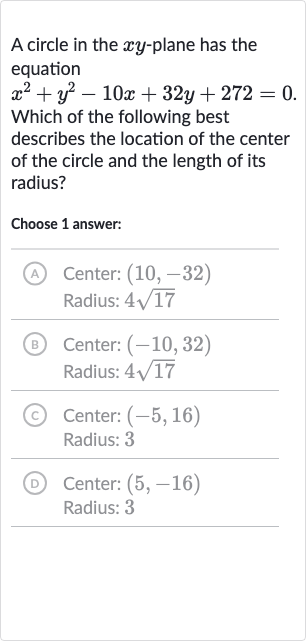
Q. A circle in the

x y

-plane has the equation

\newline

x^{2}+y^{2}-10 x+32 y+272=0 \text {. }

\newline

Which of the following best describes the location of the center of the circle and the length of its radius?

\newline

Choose

1

answer:

\newline

(A) Center:

(10,-32)

\newline

Radius:

4 \sqrt{17}

\newline

(B) Center:

(-10,32)

\newline

Radius:

4 \sqrt{17}

\newline

(-5,16)

\newline

Radius:

3

\newline

(D) Center:

(5,-16)

\newline

Radius:

3

Start Completing the Square: Write the given equation of the circle and start completing the square for the $x$ and $y$ terms. $\newline$ The given equation is $x^2 + y^2 - 10x + 32y + 272 = 0$ . $\newline$ To complete the square for the $x$ terms, we need to find the value that makes $x^2 - 10x$ into a perfect square trinomial. $\newline$ The value needed is $(10/2)^2 = 25$ . $\newline$ Similarly, for the $y$ terms, we need to find the value that makes $y^2 + 32y$ into a perfect square trinomial. $\newline$ The value needed is $(32/2)^2 = 256$ .
Add Necessary Values: Add and subtract the necessary values to complete the square inside the equation. $\newline$ We add $25$ to both sides for the $x$ terms and $256$ to both sides for the $y$ terms, and subtract these values outside the completed squares to keep the equation balanced. $\newline$ The equation becomes $x^2 - 10x + 25 + y^2 + 32y + 256 = 272 + 25 + 256$ .
Simplify the Equation: Simplify the equation by combining like terms and writing the completed squares. $\newline$ The equation now is $(x^2 - 10x + 25) + (y^2 + 32y + 256) = 553$ . $\newline$ This simplifies to $(x - 5)^2 + (y + 16)^2 = 553$ .
Compare to Standard Form: Compare the simplified equation to the standard form of a circle's equation. $\newline$ The standard form of a circle's equation is $(x - h)^2 + (y - k)^2 = r^2$ , where $(h, k)$ is the center and $r$ is the radius. $\newline$ From our equation $(x - 5)^2 + (y + 16)^2 = 553$ , we can see that the center $(h, k)$ is $(5, -16)$ and $r^2$ is $553$ .
Calculate the Radius: Calculate the radius of the circle. $\newline$ To find the radius $r$ , we take the square root of $553$ . $\newline$ The radius $r$ is $\sqrt{553}$ . $\newline$ However, $553$ is not a perfect square, so we need to simplify the square root. $\newline$ $553 = 17 \times 32 + 9 = 17 \times 33$ . $\newline$ So, the radius $r$ is $\sqrt{17 \times 33} = \sqrt{17} \times \sqrt{33}$ . $\newline$ Since $\sqrt{33}$ is not an integer, we cannot simplify further, and the radius remains $\sqrt{553}$ .
Identify Correct Answer: Identify the correct answer from the given options. $\newline$ The center of the circle is $(5, -16)$ , and the radius is $\sqrt{553}$ . $\newline$ None of the given options match this radius, so there must be a mistake in the calculation of the radius.