Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

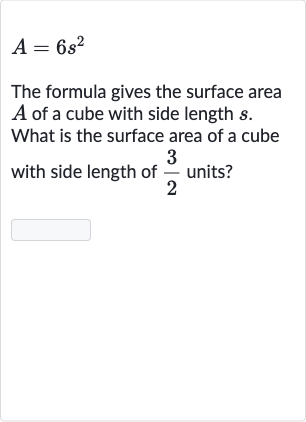
A=6s^(2) The formula gives the surface area A of a cube with side length s. What is the surface area of a cube with side length of (3)/(2) units?

A=6s2 A=6 s^{2} \newlineThe formula gives the surface area A A of a cube with side length s s . What is the surface area of a cube with side length of 32 \frac{3}{2} units?

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Precalculusright chevron icon
Find trigonometric ratios using a Pythagorean or reciprocal identityright chevron icon

Full solution

Q. A=6s2 A=6 s^{2} \newlineThe formula gives the surface area A A of a cube with side length s s . What is the surface area of a cube with side length of 32 \frac{3}{2} units?
  1. Identify formula for surface area: Identify the formula for the surface area of a cube.\newlineThe formula for the surface area of a cube is A=6s2A = 6s^2, where ss is the side length of the cube.
  2. Substitute given side length: Substitute the given side length into the formula.\newlineThe given side length is s=32 s = \frac{3}{2} units. Substitute this value into the formula to find the surface area.\newlineA=6×(32)2 A = 6 \times \left(\frac{3}{2}\right)^2
  3. Calculate square of side length: Calculate the square of the side length.\newline(32)2=(32)×(32)=94(\frac{3}{2})^2 = (\frac{3}{2}) \times (\frac{3}{2}) = \frac{9}{4}
  4. Multiply by 66 for surface area: Multiply the result by 66 to find the surface area.\newlineA=6×(94)A = 6 \times \left(\frac{9}{4}\right)\newlineA=544A = \frac{54}{4}
  5. Simplify fraction for final area: Simplify the fraction to get the final surface area. \newline544\frac{54}{4} can be simplified by dividing both the numerator and the denominator by 22.\newlineA=272A = \frac{27}{2}

More problems from Find trigonometric ratios using a Pythagorean or reciprocal identity

Question
If cos⁡(θ)=817 \cos(\theta)=\frac{8}{17} and 0∘<θ<90∘ 0^\circ<\theta<90^\circ , what is sec⁡(θ) \sec(\theta) ? Write your answer in simplified, rationalized form. sec⁡(θ)= \sec(\theta)= ______
Get tutor helpright-arrow

Posted 5 months ago

Question
cos⁡(x)=−0.3\cos(x) = -0.3. What is sin⁡(90∘−x)\sin(90^\circ - x)? ____
Get tutor helpright-arrow

Posted 8 months ago

Question
If csc⁡(θ)=178 \csc(\theta) = \frac{17}{8} and 0∘<θ<90∘ 0^\circ < \theta < 90^\circ , what is tan⁡(θ) \tan(\theta) ?\newlineWrite your answer in simplified, rationalized form.\newlinetan⁡(θ)= \tan(\theta) = ______\newline
Get tutor helpright-arrow

Posted 8 months ago

Question
Is the sine function even or odd?\newlineChoices:\newline[A]even\text{[A]even}\newline[B]odd\text{[B]odd}
Get tutor helpright-arrow

Posted 8 months ago

Question
Write the sentence as an equation. \newline8989 is equal to the quotient of yy and 1717 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 7 months ago

Question
Write the sentence as an equation. \newline4545 is equal to the quotient of zz and 55 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for hh. \newline h2+24h=0h^2 + 24h = 0 \newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas. \newline h=h = _____
Get tutor helpright-arrow

Posted 9 months ago

Question
h2−4h=0h^2 - 4h = 0
Get tutor helpright-arrow

Posted 7 months ago

Question
Combine the like terms to create an equivalent expression:\newline−k−(−8k)=□-k-(-8k)=\square
Get tutor helpright-arrow

Posted 10 months ago

Question
Which of the following degree measures is equal to 5π 5 \pi radians?\newline(The number of degrees of arc in a circle is 360360 . The number of radians of arc in a circle is 2π 2 \pi .)\newlineChoose 11 answer:\newline(A) 144∘ 144^{\circ} \newline(B) 900∘ 900^{\circ} \newline(C) 1,080∘ 1,080^{\circ} \newline(D) 1,800∘ 1,800^{\circ}
Get tutor helpright-arrow

Posted 7 months ago

View all (56)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant