Full solution
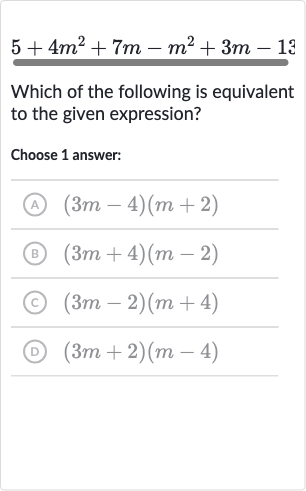
Q. Which of the following is equivalent to the given expression?Choose answer:(A) (B) (C) (D)
- Combine Like Terms: Combine like terms in the expression . We combine the terms: . We combine the terms: . We combine the constant terms: . The simplified expression is .
- Factor Quadratic Expression: Factor the quadratic expression . We look for two numbers that multiply to and add to . The numbers and satisfy these conditions because and . We rewrite the middle term using these numbers: .
- Group and Factor: Group the terms to factor by grouping: . Factor out the common factor from each group: .
- Factor Common Binomial: Factor out the common binomial factor : .This is the factored form of the expression.