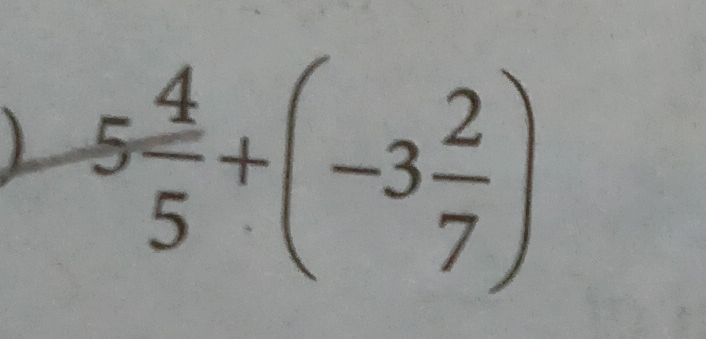AI tutor
Full solution
Q.
- Simplify First Term: Simplify the first term . The in the numerator and the in the denominator will cancel each other out because any number divided by itself is . So, .
- Simplify Second Term: Simplify the second term . This is a multiplication of and . So, .
- Add Results: Add the results from Step and Step .We have from the first term and from the second term.So, .To add these, we need a common denominator. The common denominator is .
- Convert to Common Denominator: Convert the whole number to a fraction with the common denominator. can be written as , which is .So, .
- Perform Subtraction: Perform the subtraction with the common denominator.Now that we have a common denominator, we can subtract the numerators..
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help