Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

4p-2t=6

8p+6t=12+2t
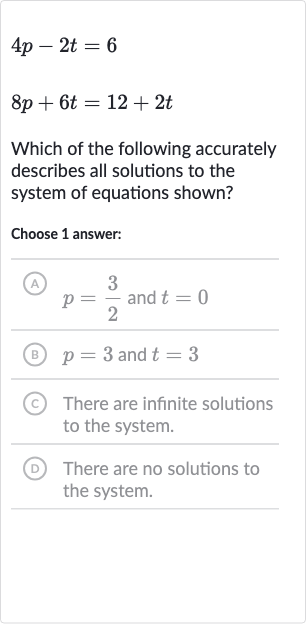
Which of the following accurately describes all solutions to the system of equations shown?
Choose 1 answer:
(A)
p=(3)/(2) and
t=0
(B)
p=3 and
t=3
(c) There are infinite solutions to the system.
(D) There are no solutions to the system.

$4 p-2 t=6$ $\newline$ $8 p+6 t=12+2 t$ $\newline$ Which of the following accurately describes all solutions to the system of equations shown? $\newline$ Choose $1$ answer: $\newline$ (A) $p=\frac{3}{2}$ and $t=0$ $\newline$ (B) $p=3$ and $t=3$ $\newline$ (C) There are infinite solutions to the system. $\newline$ (D) There are no solutions to the system.

View step-by-step help

View step-by-step help

Solve rational equations

Full solution

Q.

4 p-2 t=6

\newline

8 p+6 t=12+2 t

\newline

Which of the following accurately describes all solutions to the system of equations shown?

\newline

Choose

1

answer:

\newline

(A)

p=\frac{3}{2}

and

t=0

\newline

(B)

p=3

and

t=3

\newline

(C) There are infinite solutions to the system.

\newline

(D) There are no solutions to the system.

Write down equations: Write down the system of equations. $\newline$ We have the following system of equations: $\newline$ $4p - 2t = 6$ $\newline$ $8p + 6t = 12 + 2t$
Simplify second equation: Simplify the second equation by subtracting $2t$ from both sides. $\newline$ $8p + 6t - 2t = 12 + 2t - 2t$ $\newline$ $8p + 4t = 12$
Compare coefficients: Compare the coefficients of the simplified system of equations. $\newline$ We now have: $\newline$ $4p - 2t = 6$ $\newline$ $8p + 4t = 12$ $\newline$ Notice that the second equation is exactly twice the first equation.
Determine solution type: Determine if the system has one solution, no solution, or infinitely many solutions. $\newline$ Since the second equation is a multiple of the first, the two equations are dependent, and the system has infinitely many solutions.

More problems from Solve rational equations

Question

Evaluate the expression for

t = -4.4

\newline

Write your answer in simplest form.

\newline

` frac{t - 4}{t - 2} =` ____

Posted 1 month ago

Question

Simplify. Write your answer using whole numbers and variables.

\newline

\frac{g}{3g^2 - 4g}

Posted 2 months ago

Question

Simplify. Express your answer as a single fraction in simplest form.

\frac{3}{2} - \frac{5p^3}{3}

Posted 2 months ago

Question

Simplify. Express your answer as a single fraction in simplest form.

\newline

\frac{1 + \frac{v^5w}{3}}{1}

\newline

Posted 2 months ago

Question

h

\newline

\frac{-5}{h - 2} = \frac{-4}{h - 3}

\newline

1

2

\newline

h =

h =

Posted 1 month ago

Question

What is the equation of the vertical asymptote of

y = \frac{1}{x + 9}

Posted 2 months ago

Question

What is the excluded value for

y = \frac{1}{x - 9} - 9

\newline

Posted 2 months ago

Question

Simplify. Write your answer using whole numbers and variables.

\newline

\frac{9k + 4}{9k^2 + 4k}

\newline

Posted 2 months ago

Question

Divide. Write your answer in simplest form.

\newline

\frac{g- 1}{10g^3 + 25g^2} \div (g- 1)

\newline

Posted 2 months ago

Question

Simplify. Express your answer as a single fraction in simplest form.

\newline

\frac{5 - \frac{3}{t^3}}{1}

\newline

Posted 2 months ago