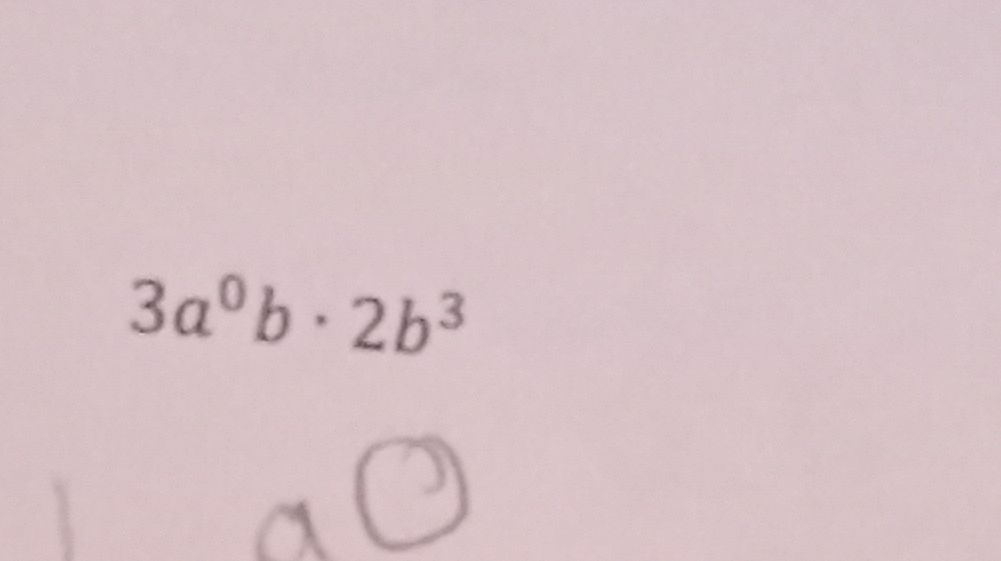Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Simplify. 3a^(0)b*2b^(3)

Simplify.\newline3a0b⋅2b3 3 a^{0} b \cdot 2 b^{3}

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Multiply radical expressionsright chevron icon

Full solution

Q. Simplify.\newline3a0b⋅2b3 3 a^{0} b \cdot 2 b^{3}
  1. Question Prompt: Question prompt: What is the simplified form of the expression 3a0b∗2b33a^{0}b\ast2b^{3}?
  2. Simplify Exponent: Simplify the term with the zero exponent, a0a^{0}. Any number raised to the power of zero is 11. Therefore, a0=1a^{0} = 1.\newlineCalculation: a0=1a^{0} = 1
  3. Multiply Terms: Multiply the simplified term from Step 11 with the remaining terms in the expression. Since a0=1a^{0} = 1, the expression becomes 3×1×b×2×b33 \times 1 \times b \times 2 \times b^{3}.\newlineCalculation: 3×1×b×2×b3=3×b×2×b33 \times 1 \times b \times 2 \times b^{3} = 3 \times b \times 2 \times b^{3}
  4. Combine Constants and Like Terms: Combine the constants and the like terms. The constants are 33 and 22, and the like terms are bb and b3b^{3}.\newlineCalculation: 3×2=63 \times 2 = 6 and b×b3=b1+3=b4b \times b^{3} = b^{1+3} = b^{4}
  5. Write Final Expression: Write the final simplified expression by multiplying the results from Step 33.\newlineCalculation: 6×b4=6b46 \times b^{4} = 6b^{4}

More problems from Multiply radical expressions

Question
Find the real-number root. \newline16\sqrt{16}\newline\newlineWrite your answer in simplified form. \newline____\_\_\_\_
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the real-number root. \newline916\sqrt{\frac{9}{16}}\newlineSimplify your answer and write it as a decimal or as a proper or improper fraction.\newline_____\_\_\_\_\_
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the real-number root using a calculator. Round your answer to the nearest thousandth. \newline`\sqrt{957} `\newline _______
Get tutor helpright-arrow

Posted 2 months ago

Question
Multiply. Write your answer in simplest form. \newline273×42\sqrt{273} \times \sqrt{42}\newline______
Get tutor helpright-arrow

Posted 1 month ago

Question
Simplify. \newline634\sqrt{\frac{63}{4}}\newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Simplify. Rationalize the denominator.\newline−49−2\frac{-4}{9 - \sqrt{2}}\newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Solve for z z .\newline16=z 16 = \sqrt{z} \newlinez= z = _____
Get tutor helpright-arrow

Posted 2 months ago

Question
Simplify the radical expression. 14x14 \sqrt{14x^{14}} Write your answer in the form A A , B \sqrt{B} , or AB A\sqrt{B} , where A A and B B are constants or expressions in x x . Use at most one radical in your answer, and at most one absolute value symbol in your expression for A A . ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Simplify the radical expression.\newline12x12\sqrt{12x^{12}}\newlineWrite your answer in the form AA, B\sqrt{B}, or ABA\sqrt{B}, where AA and BB are constants or expressions in xx. Use at most one radical in your answer, and at most one absolute value symbol in your expression for AA.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 2 months ago

Question
What is the value of sin⁡(5π6)? \sin \left(\frac{5 \pi}{6}\right) ? \newlineChoose 11 answer:\newline(A) −32 -\frac{\sqrt{3}}{2} \newline(B) −22 -\frac{\sqrt{2}}{2} \newline(C) 12 \frac{1}{2} \newline(D) 150150
Get tutor helpright-arrow

Posted 4 months ago

View all (18)