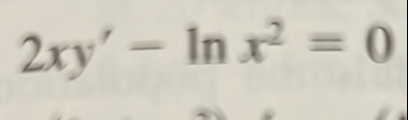AI tutor
Full solution
Q.
- Isolate derivative : Given the differential equation , we want to find the general solution . Let's start by isolating the derivative .
- Simplify : Now, we notice that can be simplified using logarithm properties.So, we can rewrite the equation as:
- Cancel 's: Simplifying the equation by canceling the 's, we get:Now we have a separable differential equation.
- Integrate both sides: To solve the separable differential equation, we integrate both sides with respect to .
- Integrate known integral: The left side of the equation integrates to . The right side is a known integral.The integral of with respect to is , where is the constant of integration.
- Find general solution: We have found the general solution of the differential equation.The general solution is .
More problems from Solve complex trigonomentric equations
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help