Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

2x^(2)+5x-k=0
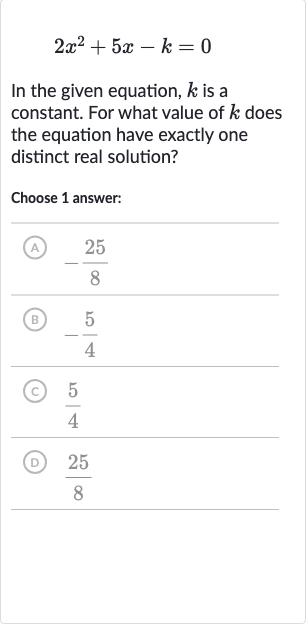
In the given equation,
k is a constant. For what value of
k does the equation have exactly one distinct real solution?
Choose 1 answer:
(A)
-(25)/(8)
(B)
-(5)/(4)
(c)
(5)/(4)
(D)
(25)/(8)

$2 x^{2}+5 x-k=0$ $\newline$ In the given equation, $k$ is a constant. For what value of $k$ does the equation have exactly one distinct real solution? $\newline$ Choose $1$ answer: $\newline$ (A) $-\frac{25}{8}$ $\newline$ (B) $-\frac{5}{4}$ $\newline$ (C) $\frac{5}{4}$ $\newline$ (D) $\frac{25}{8}$

View step-by-step help

View step-by-step help

Domain and range

Full solution

Q.

2 x^{2}+5 x-k=0

\newline

In the given equation,

k

is a constant. For what value of

k

does the equation have exactly one distinct real solution?

\newline

Choose

1

answer:

\newline

(A)

-\frac{25}{8}

\newline

(B)

-\frac{5}{4}

\newline

(C)

\frac{5}{4}

\newline

(D)

\frac{25}{8}

Quadratic Equation Discriminant: A quadratic equation $ax^2 + bx + c = 0$ has exactly one distinct real solution when its discriminant $b^2 - 4ac$ is equal to zero. This is because the discriminant determines the nature of the roots of the quadratic equation.
Given Equation and Variables: For the given equation $2x^2 + 5x - k = 0$ , $a = 2$ , $b = 5$ , and $c = -k$ . We will set the discriminant equal to zero and solve for $k$ .
Discriminant: $b^2 - 4ac = 0$
Substitute Values: Substitute the values of $a$ , $b$ , and $c$ into the discriminant equation: $\newline$ $(5)^2 - 4(2)(-k) = 0$
Simplify Equation: Simplify the equation: $25 + 8k = 0$
Solve for k: Solve for k: $\newline$ $8k = -25$ $\newline$ $k = -\frac{25}{8}$
Final Solution: The value of $k$ for which the equation has exactly one distinct real solution is $-\frac{25}{8}$ .

More problems from Domain and range

Question

What is the domain of this function?

\newline

(9,–2)(6,–10)(–3,9)(5,2)

\newline

\newline

(A)\{–3,5,6,9\}\newline(B)\{–10,–2,2,9\}\newline(C)\{2,5,6,9\}\newline(D)\{–6,–3,5,9\}

Posted 1 year ago

Question

Look at this set of ordered pairs:

\newline

(2, 14)

\newline

(10, 12)

\newline

(3, -19)

\newline

Is this relation a function?

\newline

\newline

\text{[[yes]}

\text{[no]]}

Posted 1 year ago

Question

Use the following function rule to find

f(11)

\newline

f(x) = -10 - 7x

\newline

f(11) =

Posted 1 year ago

Question

Find the slope of the line

y = -3x - \frac{2}{5}

\newline

Simplify your answer and write it as a proper fraction, improper fraction, or integer.

\newline

\_\_\_\_\_

Posted 1 year ago

Question

A line has a slope of

3

and passes through the point

(-2,-10)

. Write its equation in slope-intercept form.

\newline

Write your answer using integers, proper fractions, and improper fractions in simplest form.

Posted 1 year ago

Question

What is the range of this function?

\newline

\newline

\newline

\text{all real numbers}

\newline

\{y \mid y \geq 0\}

\newline

\{y \mid y \leq 0\}

\newline

\{y \mid y > 0\}

Posted 1 year ago

Question

g(x)

g(x)

is the translation

1

f(x) = |x|

\newline

Write your answer in the form

a|x - h| + k

a

h

k

\newline

g(x) =

\newline

Posted 1 year ago

Question

What is the domain of this function?

\newline

(8,–1)(7,–11)(–4,10)(4,3)

\newline

\newline

(A)\{–4,4,7,8\}\newline

(B)\{–11,–1,3,10\}\newline

(C)\{3,4,7,8\}\newline

(D)\{–7,–4,4,8\}

Posted 1 year ago

Question

What is the domain of this function?

\newline

(10,\,–3)(2,\,–9)(–5,\,8)(3,\,1)

\newline

\newline

[\{–5,2,3,10\}][\{–9,\,–3,1,8\}][\{1,2,3,10\}][\{–10,\,–5,2,3\}]

Posted 1 year ago

Question

What is the domain of this function?

\newline

(1,–4)(9,–12)(–6,11)(7,5)

\newline

\newline\\(A)\{–6,1,7,9\}\newline

(B)\{–12,–4,5,11\}\newline

(C\{5,1,7,9\}\newline

(D)\{–9,–6,1,7\}

Posted 1 year ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant