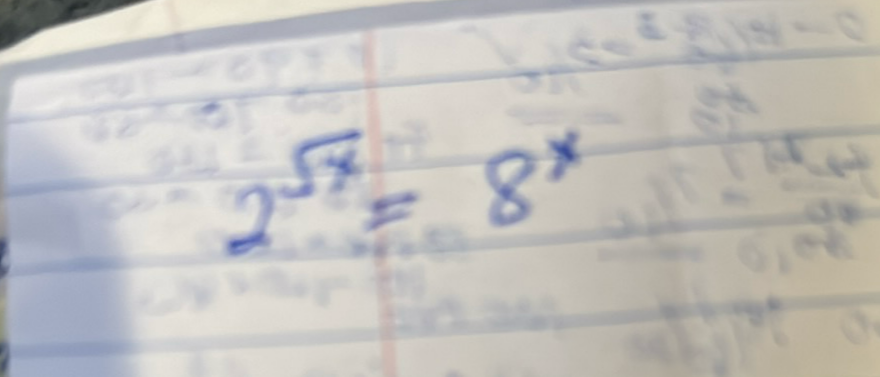$2^{\sqrt{x}}=8^{x}$ .Find `x`

View step-by-step help

Home

Math Problems

Algebra 2

Simplify radical expressions involving fractions

Full solution

2^{\sqrt{x}}=8^{x}

.Find `x`

Recognize Power of $2$ : We start by recognizing that $8$ is a power of $2$ , specifically $8 = 2^3$ . We can use this to rewrite the equation in terms of the same base. $\newline$ $2^{\sqrt{x}} = (2^3)^{x}$
Apply Power Rule: Now we apply the power rule of exponents, which states that $(a^b)^c = a^{b*c}$ . So we can rewrite the right side of the equation as: $2^{\sqrt{x}} = 2^{3x}$
Set Exponents Equal: Since the bases are the same and the equation is an equality, we can set the exponents equal to each other: $\sqrt{x} = 3x$
Square Both Sides: To solve for $x$ , we can square both sides of the equation to get rid of the square root: $(\sqrt{x})^2 = (3x)^2$ $x = 9x^2$
Solve Quadratic Equation: We now have a quadratic equation. To solve for $x$ , we need to set the equation to zero: $0 = 9x^2 - x$
Factorize Equation: This is a quadratic equation in standard form. We can solve for $x$ by factoring or using the quadratic formula. However, this equation can be factored easily: $\newline$ $0 = x(9x - 1)$
Find Potential Solutions: Setting each factor equal to zero gives us two possible solutions for $x$ : $x = 0$ or $9x - 1 = 0$
Check $x = 0$ : Solving the second equation for $x$ gives us: $\newline$ $9x = 1$ $\newline$ $x = \frac{1}{9}$
Check $x = \frac{1}{9}$ : We now have two potential solutions: $x = 0$ and $x = \frac{1}{9}$ . However, we must check these solutions in the original equation because squaring both sides could have introduced an extraneous solution.
Check $x = \frac{1}{9}$ : We now have two potential solutions: $x = 0$ and $x = \frac{1}{9}$ . However, we must check these solutions in the original equation because squaring both sides could have introduced an extraneous solution.First, we check $x = 0$ in the original equation: $\newline$ $2^{\sqrt{0}} = 8^{0}$ $\newline$ $2^0 = 1$ $\newline$ $1 = 1$ $\newline$ This solution checks out.
Check $x = \frac{1}{9}$ : We now have two potential solutions: $x = 0$ and $x = \frac{1}{9}$ . However, we must check these solutions in the original equation because squaring both sides could have introduced an extraneous solution.First, we check $x = 0$ in the original equation: $\newline$ $2^{\sqrt{0}} = 8^{0}$ $\newline$ $2^0 = 1$ $\newline$ $1 = 1$ $\newline$ This solution checks out.Now, we check $x = \frac{1}{9}$ in the original equation: $\newline$ $2^{\sqrt{\frac{1}{9}}} = 8^{\frac{1}{9}}$ $\newline$ $2^{\frac{1}{3}} = (2^3)^{\frac{1}{9}}$ $\newline$ $x = 0$ $0$ $\newline$ $x = 0$ $1$ $\newline$ This solution also checks out.