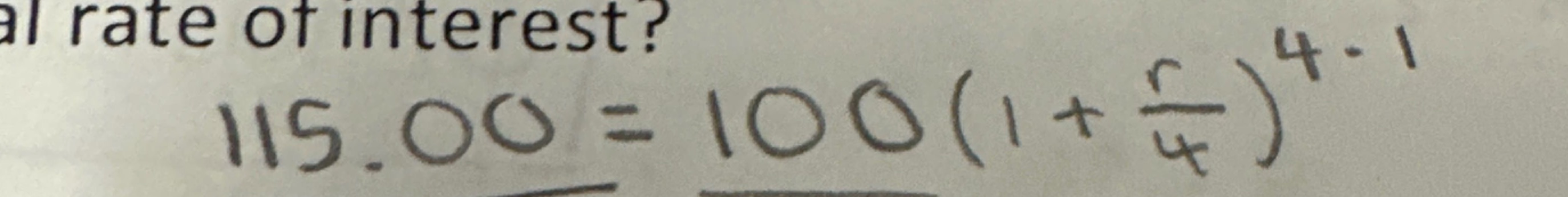AI tutor
Full solution
Q. Find the rate of interest.
- Simplify exponent: To solve for the interest rate , we need to isolate in the equation . First, we simplify the exponent on the right side of the equation.
- Divide by : The exponent simplifies to . So, the equation becomes .
- Take cube root: Next, we divide both sides of the equation by to isolate the term with on one side. This gives us .
- Subtract : Performing the division, we get .
- Isolate : Now, we need to take the cube root of both sides to remove the exponent on the right side. The cube root of is approximately .
- Multiply by : After taking the cube root, we have .
- Convert to percentage: Subtract from both sides to isolate on the right side. This gives us .
- Convert to percentage: Subtract from both sides to isolate on the right side. This gives us .Finally, multiply both sides by to solve for . This gives us .
- Convert to percentage: Subtract from both sides to isolate on the right side. This gives us .Finally, multiply both sides by to solve for . This gives us .Multiplying by gives us .
- Convert to percentage: Subtract from both sides to isolate on the right side. This gives us .Finally, multiply both sides by to solve for . This gives us .Multiplying by gives us .To express as a percentage, we multiply by . So, .
- Convert to percentage: Subtract from both sides to isolate on the right side. This gives us .Finally, multiply both sides by to solve for . This gives us .Multiplying by gives us .To express as a percentage, we multiply by . So, .Multiplying by gives us .
More problems from Find trigonometric functions using a calculator
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help