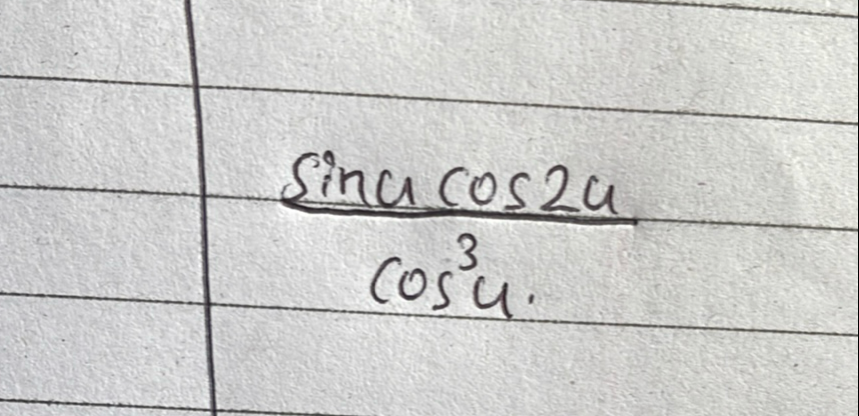Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

(sin u cos 2u)/(cos^(3)u)

sin⁡ucos⁡2ucos⁡3u \frac{\sin u \cos 2 u}{\cos ^{3} u}

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Sin, cos, and tan of special anglesright chevron icon

Full solution

Q. sin⁡ucos⁡2ucos⁡3u \frac{\sin u \cos 2 u}{\cos ^{3} u}
  1. Apply double angle formula: Apply the double angle formula for cosine.\newlineThe double angle formula for cosine is cos⁡(2u)=cos⁡2(u)−sin⁡2(u)\cos(2u) = \cos^2(u) - \sin^2(u).
  2. Substitute formula into expression: Substitute the double angle formula into the original expression.\newlineReplace cos⁡(2u)\cos(2u) in the original expression with (cos⁡2(u)−sin⁡2(u))(\cos^2(u) - \sin^2(u)) to get sin⁡u⋅(cos⁡2(u)−sin⁡2(u))cos⁡3u\frac{\sin u \cdot (\cos^2(u) - \sin^2(u))}{\cos^{3}u}.
  3. Distribute sin⁡u\sin u: Distribute sin⁡u\sin u over the terms inside the parentheses. Multiply sin⁡u\sin u with both cos⁡2(u)\cos^2(u) and −sin⁡2(u)-\sin^2(u) to get (sin⁡u⋅cos⁡2(u)−sin⁡3(u))/(cos⁡3u)(\sin u \cdot \cos^2(u) - \sin^3(u))/(\cos^{3}u).
  4. Split fraction into two: Split the fractions" target="_blank" class="backlink">fraction into two separate fractions. Divide both terms in the numerator by cos⁡3u\cos^{3}u to get (sin⁡u⋅cos⁡2(u))/(cos⁡3u)−(sin⁡3(u))/(cos⁡3u)(\sin u \cdot \cos^2(u))/(\cos^{3}u) - (\sin^3(u))/(\cos^{3}u).
  5. Simplify the fractions: Simplify the fractions.\newlineThe first term simplifies to sin⁡u/cos⁡u\sin u/\cos u because one cos⁡u\cos u in the numerator and denominator cancels out. The second term simplifies to −sin⁡3(u)/cos⁡3(u)-\sin^3(u)/\cos^3(u), which is −tan⁡3(u)-\tan^3(u).
  6. Combine simplified terms: Combine the simplified terms. The expression now is tan⁡u−tan⁡3(u)\tan u - \tan^3(u).

More problems from Sin, cos, and tan of special angles

Question
Find all solutions with 0∘≤θ≤180∘0^\circ \leq \theta \leq 180^\circ. Give the exact answer(s) in simplest form. If there are multiple answers, separate them with commas.\newlinecos⁡(θ)=−1\cos(\theta) = -1\newline‾∘\underline{\hspace{2em}}^\circ
Get tutor helpright-arrow

Posted 7 months ago

Question
Kimi's school is 1515 kilometers west of her house and 88 kilometers south of her friend Franklin's house. Every day, Kimi bicycles from her house to her school. After school, she bicycles from her school to Franklin's house. Before dinner, she bicycles home on a bike path that goes straight from Franklin's house to her own house. How far does Kimi bicycle each day?\newline_____\_\_\_\_\_ kilometers
Get tutor helpright-arrow

Posted 7 months ago

Question
Evaluate. Write your answer as an integer or as a decimal rounded to the nearest hundredth.\newlinecos⁡35∘=\cos 35^\circ = __
Get tutor helpright-arrow

Posted 7 months ago

Question
Find all solutions with −90∘≤θ≤90∘-90^\circ \leq \theta \leq 90^\circ. Give the exact answer(s) in simplest form. If there are multiple answers, separate them with commas.\newlinecsc⁡(θ)=−1\csc(\theta) = -1\newline____ ∘\_\_\_\_\,^\circ
Get tutor helpright-arrow

Posted 8 months ago

Question
Evaluate. Write your answer in simplified, rationalized form. Do not round.\newlinecot⁡30∘=\cot 30^\circ = ______
Get tutor helpright-arrow

Posted 7 months ago

Question
The terminal side of an angle θ\theta in standard position intersects the unit circle at (8485,1385)(\frac{84}{85}, \frac{13}{85}). What is cos⁡(θ)\cos(\theta)?\newlineWrite your answer in simplified, rationalized form.\newline______
Get tutor helpright-arrow

Posted 7 months ago

Question
−90∘<θ<90∘-90^\circ < \theta < 90^\circ. Find the value of θ\theta in degrees.\newlinetan⁡(θ)=0\tan(\theta) = 0\newlineWrite your answer in simplified, rationalized form. Do not round.\newlineθ=\theta = ____∘^\circ\newline
Get tutor helpright-arrow

Posted 8 months ago

Question
Evaluate. Write your answer in simplified, rationalized form. Do not round.\newlinecsc⁡60∘=\csc 60^\circ = ______
Get tutor helpright-arrow

Posted 8 months ago

Question
θ\theta is an acute angle. Find the value of θ\theta in degrees.\newlinesec⁡(θ)=2\sec(\theta) = \sqrt{2}\newlineθ=\theta = ____°\degree
Get tutor helpright-arrow

Posted 7 months ago

Question
Evaluate. Write your answer in simplified, rationalized form. Do not round.\newlinecos⁡90∘=\cos 90^\circ = ____
Get tutor helpright-arrow

Posted 9 months ago

View all (90)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant