Full solution
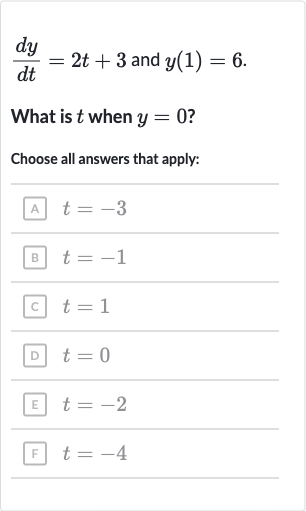
Q. What is when ?Choose all answers that apply:(A) (B) (C) (D) (E) (F)
- Integrate and Find Solution: Given the differential equation and the initial condition , we need to integrate the differential equation to find the general solution for .
- Use Initial Condition: Integrate with respect to to find ., where is the constant of integration.
- Substitute Constant and Solve: Use the initial condition to find the value of the constant .
- Find when : Substitute the value of back into the general solution to get the particular solution for .
- Factor and Solve Quadratic Equation: Now we need to find the value of when .We have a quadratic equation to solve: .
- Identify Values of : Factor the quadratic equation.
- Identify Values of : Factor the quadratic equation.Set each factor equal to zero and solve for .
- Identify Values of t: Factor the quadratic equation. Set each factor equal to zero and solve for t. We have found two values of t when : and . These correspond to options B and E.
More problems from Solve complex trigonomentric equations
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help