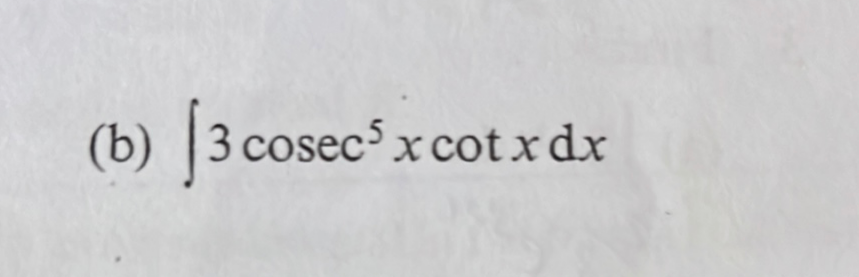AI tutor
Full solution
Q.
- Recognize Trigonometric Functions: Given the integral to solve is . We can start by recognizing that is and is . So, we rewrite the integral in terms of sine and cosine: Now, let's use a substitution method where we let , which means .
- Perform Substitution: Perform the substitution:The integral becomes:
- Integrate with Respect to u: Now we integrate with respect to : The antiderivative of is .So, we have , where is the constant of integration.
- Substitute Back for u: Substitute back for u to get the answer in terms of x:Simplify the expression:This is our final answer.
More problems from Solve complex trigonomentric equations
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help