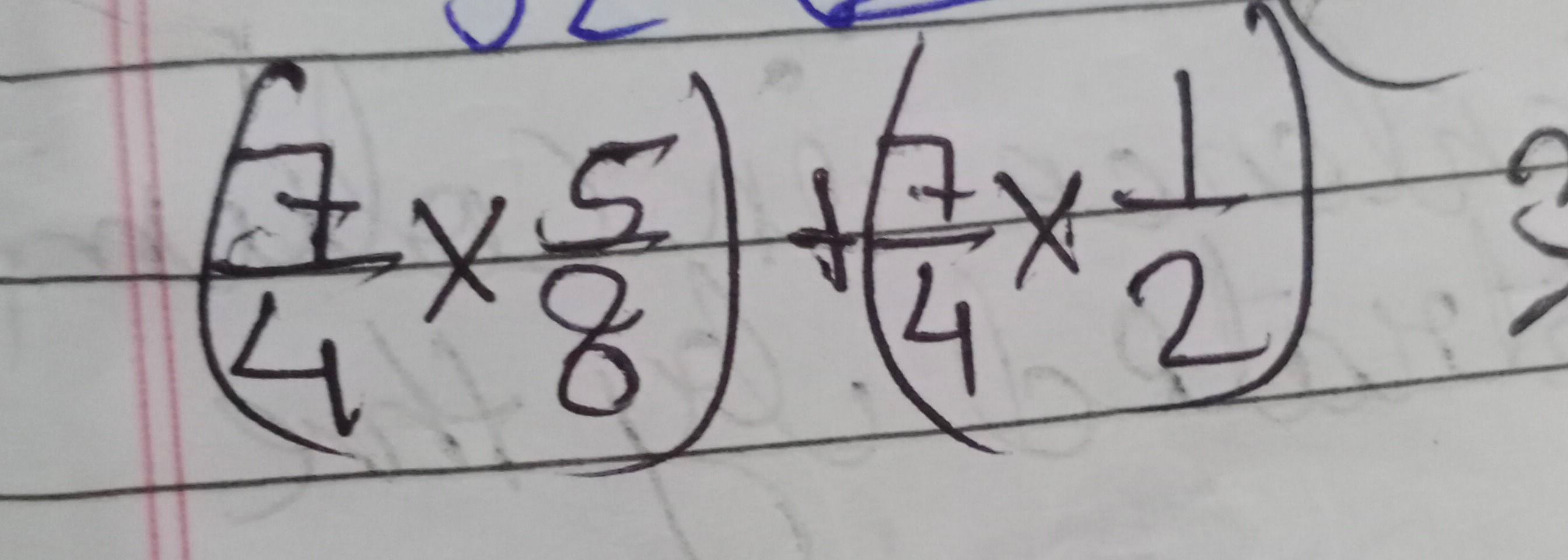AI tutor
Full solution
Q.
- Simplify First Multiplication: We will first simplify the multiplication within each fractions" target="_blank" class="backlink">fraction separately.The first multiplication is .To multiply fractions, we multiply the numerators together and the denominators together.So, .
- Simplify Second Multiplication: Now we will simplify the second multiplication, which is . Again, we multiply the numerators together and the denominators together. So, .
- Add Fractions: Next, we will add the two results together.We have from the first multiplication and from the second multiplication.To add fractions, we need a common denominator.The common denominator for and is .
- Convert to Common Denominator: We will convert to have the common denominator of . To do this, we multiply both the numerator and the denominator by . So, becomes .
- Add Fractions with Common Denominator: Now we can add the two fractions with the common denominator.So, .
- Add Numerators: We add the numerators together. . So, the combined fraction is .
- Check for Simplest Form: The fraction is already in its simplest form because and have no common factors other than .Therefore, the simplified result of the expression is .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help