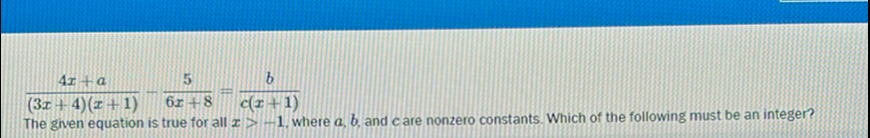
(4x+a)/((3x+4)(x+1))-(5)/(6x+8)=(b)/(c(x+1)) The given equation is true for all x -1, where a,b, and c are nonzero constants. Which of the following must be an integer?

Question

(4x+a)/((3x+4)(x+1))-(5)/(6x+8)=(b)/(c(x+1)) The given equation is true for all  x > -1, where  a,b, and  c are nonzero constants. Which of the following must be an integer?

Bytelearn · Accepted Answer

Simplify Equation: First, let's simplify the equation by combining the terms on the left side over a common denominator.Common Denominator: The common denominator for the terms on the left side is (3x+4)(x+1). We can rewrite the second term on the left side to have this common denominator by multiplying the numerator and denominator by (x+1)/2, since 6x+8 = 2(3x+4).Rewrite Equation: Now, let's rewrite the equation with the common denominator: (4x+a)/((3x+4)(x+1)) - (5(x+1))/(2(3x+4)(x+1)) = (b)/(c(x+1))Combine Left Side: Combine the terms on the left side: [(4x+a) - (5(x+1)/2)]/((3x+4)(x+1)) = (b)/(c(x+1))Distribute -5/2: Distribute the -5/2 across the (x+1) in the numerator: [(4x+a) - (5x/2 + 5/2)]/((3x+4)(x+1)) = (b)/(c(x+1))Combine Like Terms: Combine like terms in the numerator: [(8x/2 + 2a/2) - (5x/2 + 5/2)]/((3x+4)(x+1)) = (b)/(c(x+1))Simplify Numerator: Simplify the numerator: [(8x - 5x)/2 + (2a - 5)/2]/((3x+4)(x+1)) = (b)/(c(x+1))Equate Numerators: Further simplify the numerator: [(3x)/2 + (2a - 5)/2]/((3x+4)(x+1)) = (b)/(c(x+1))Clear Fraction: Since the equation is true for all x > -1, the numerators on both sides of the equation must be equal when the denominators are the same. Therefore, we can equate the numerators: (3x)/2 + (2a - 5)/2 = bCancel Variable Term: Multiply both sides by 2 to clear the fraction: 3x + 2a - 5 = 2bSince 3x is a variable term and 2b is a constant term, for the equation to hold true for all x > -1, the variable terms must cancel out. This means that the coefficient of x on the left side must be zero or the coefficient of x on the right side must be a multiple of 3. However, there is no x term on the right side, so the coefficient of x on the left must be zero. This is not possible, so there must be a mistake in the previous steps.

$\frac{4 x+a}{(3 x+4)(x+1)}-\frac{5}{6 x+8}=\frac{b}{c(x+1)}$ $\newline$ The given equation is true for all x>-1 , where $a, b$ , and $c$ are nonzero constants. Which of the following must be an integer?

Full solution

More problems from Power rule

Related topics

4x+a(3x+4)(x+1)−56x+8=bc(x+1) \frac{4 x+a}{(3 x+4)(x+1)}-\frac{5}{6 x+8}=\frac{b}{c(x+1)} (3x+4)(x+1)4x+a​−6x+85​=c(x+1)b​\newlineThe given equation is true for all x>-1 , where a,b a, b a,b, and c c c are nonzero constants. Which of the following must be an integer?

$\frac{4 x+a}{(3 x+4)(x+1)}-\frac{5}{6 x+8}=\frac{b}{c(x+1)}$ $\newline$ The given equation is true for all x>-1 , where $a, b$ , and $c$ are nonzero constants. Which of the following must be an integer?