Full solution
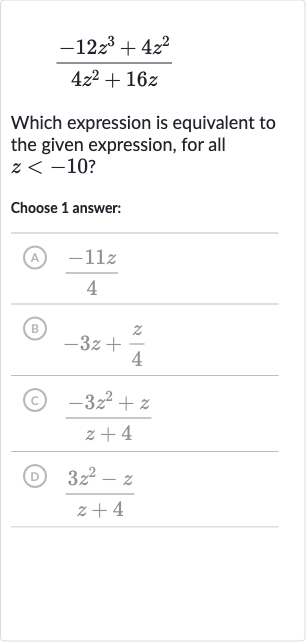
Q. Which expression is equivalent to the given expression, for all ?Choose answer:(A) (B) (C) (D)
- Simplify Expression: First, simplify the given expression: . Factor out the common terms in the numerator and the denominator.Numerator: Denominator:
- Cancel Common Factor: Next, simplify the expression by canceling out the common factor of in the numerator and the denominator.Simplified expression:
- Match Simplified Expression: Now, analyze the options given to match the simplified expression.(A) does not match the simplified expression.(B) does not match the simplified expression.(C) does not match the simplified expression.(D) matches the simplified expression.