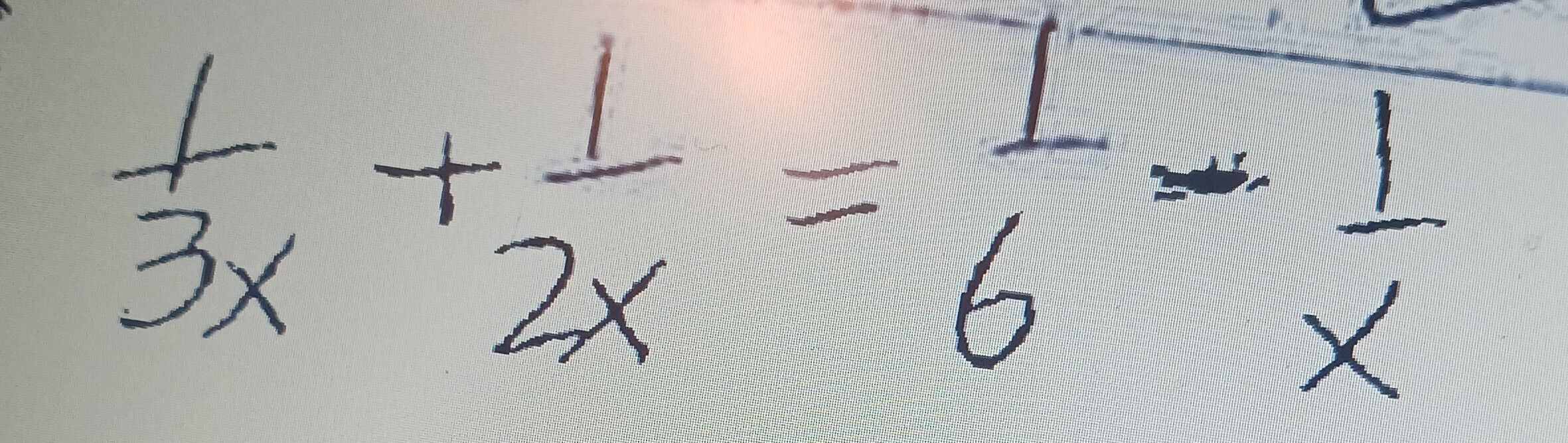AI tutor
Full solution
Q.
- Find Common Denominator: Find a common denominator for the fractions on the left side of the equation.The common denominator for and is .
- Rewrite Fractions: Rewrite each fraction on the left side with the common denominator. becomes because . becomes because .
- Add Fractions: Add the fractions on the left side..
- Rewrite Equation: Rewrite the equation with the combined fraction on the left side. rac{5}{6x} = rac{1}{6} - rac{1}{x}.
- Find Common Denominator: Find a common denominator for the fractions on the right side of the equation.The common denominator for and is .
- Rewrite Fractions: Rewrite each fraction on the right side with the common denominator. becomes because . becomes because .
- Subtract Fractions: Subtract the fractions on the right side. .
- Set Fractions Equal: Set the combined fractions from both sides equal to each other. .
- Solve for x: Since the denominators are the same, we can set the numerators equal to each other. .
- Solve for x: Since the denominators are the same, we can set the numerators equal to each other. .Solve for x.Add to both sides of the equation: ..
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help